A microphone is well known as a useful means of detecting sound waves and visualizing sound fields. For a microphone array far away from the sound source, we can extract information on its location using differences in arrival times. The accuracy of estimating a location depends mainly on the bandwidth and sensing ability of the microphone used, and the use of an instrument-grade condenser type microphone is a good example of enhancing detection ability. However, this electrical microphone array still has a problem in that it is difficult to build a sensor network and the microphone is weak in the presence of electromagnetic noise. As an alternative, fiber-based optical sensors using the acousto-optic effect have been studied with the advantage of using fiber as the sensor itself as well as the signal transmission medium. This optical sensor provides extremely wide bandwidth and long transmission due to low optical loss [1] and can operate well and safely in harsh environments like at sea or in oil wells since there is no current in the sensing unit [2].

Optical sensors to detect sound usually use optical interferometry such as Fabry-Perot, Sagnac, Michelson, and Mach-Zehnder [3-6]. Recently, a number of distributed position detection methods over a long distance (around 60 km) using a double Mach-Zehnder interferometer (MZI) have been reported [7, 8]. These studies mainly focused on detection of possible events over a long distance fiber based on acousto-optic sensing. In our previous research, we introduced a position estimation process called the received signal strength indicator method using optical acoustic sensors [9].

In this paper, we propose an array of three optical sensors using the acousto-optic effect to detect the location of a sound wave source. Each optical sensor is based on MZI and a piece of one fiber arm is used as a sensing part. To enhance the acousto-optic effect, the fiber chiefly exposed to the sound is surrounded by a membrane with a cork support. By calculating the distance-dependent arrival time of a sound signal at each sensor, the direction of the location could be deduced. This estimation might be superior to intensity-based position detection that suffers from unwanted noise caused by optical power reflected from other objects.

The conventional MZI is shown in Fig. 1. The output intensity of the MZI (coupling ratio, 50:50), I_MZ, can be expressed as

where I_in is the input light intensity of the MZI and θ(t) is the phase difference between the sensing and reference arms induced by the pressure of an acoustic wave. The phase of a light wave θ(t) can be expressed as

where β(t) is the propagation constant and L is the propagation length of a light wave. Following the definition of propagation constant, the total change in the propagation constant ∆β(t) caused by the acoustic pressure is given by

where λ is the wavelength of light, ω_a is the angular frequency of the acoustic wave, and ∆n means the change in the refractive index related to strain-optic effect, fiber strain, and stress caused by the acoustic pressure [10, 11].

Figure 2 shows the pressure-induced refractive index of fiber exposed to an acoustic wave. By using Eq. (3), propagation constants of the sensing arm β_S(t) and reference arm β_R(t) at time t can be determined as

where

As shown in Fig. 1, a part of the sensing arm with length L_S is exposed to the acoustic wave. Let L be the length of the arm in the MZI, then the phase of the light along the x-axis can be calculated as

where is used instead of β(t) considering the propagation delay along the x-axis.

From Eqs. (5) and (7), the phase difference ∆θ(t) between the two arms is given by

where

L_x is the length of the sensing arm excluding the length of sensing part L_s, c is the speed of light in free space, and θ₀ is the initial phase difference between the two arms of the MZI . By substituting Eq. (8) into Eq. (1), I_MZ can be expressed as

Since ω_anL_s/2c closes to zero in the audible frequency range (20~20 kHz), ∆γ becomes approximately ∆β_ampL_s by using sin(y) ≅ y when y approaches zero. Therefore, Eq. (10) can be approximately expressed as

From Eq. (11), if we take ∆β_ampL_s close to zero and adjust θ₀ close to , the interfered signal follows the acoustic wave applied. These conditions could be satisfied by adjusting the lengths of the two arms and the sensing part. The amplitude of the frequency components is proportional to ∆β_ampL_s. In our experiment, we used a small piece of sensing fiber and increased ∆β_amp by using the membranes attached to both sides of the sensing fiber.

We can use time difference of arrival (TDOA) to detect the location of a sound source. Figure 3 illustrates the relationship between the distance of each sensor from the source and the angle related to different arrival times. To use the plane wave model, we assume that the distance between the sensors is much shorter than that between the sensor array and the sound source. The angle θ related to the distance difference d from the source and the length x between two sensors is given by

where v is the speed of the wave, x is the distance between two sensors, and T_ij is the time delay between i^th and j^th sensor located at coordinates (x_i, y_i) and (x_j, y_j). This angle is the slope of the line intersecting the midpoint between the two sensors, as shown Fig. 3(b). The error by taking the midpoint rather than the sensor position can be ignored under the assumption that the source is far away from the array. From the angle θ, a straight line is given by

where

This line points out the position of the sound source estimated by the i^th and j^th sensors. Conclusively, two lines are obtained from two angles between the 1^st and 2^nd sensors (Line 12) and 1^st and 3^rd sensors (Line 13). The crossing point of these two lines is estimated as the position of the sound source.

The optical sensor constructed is depicted in Fig. 4. To increase the refractive index change induced by the acoustic wave, a fiber with a diameter of a few hundred microns was wound with 5 turns. Two membranes acting as the diaphragm used in a conventional electrical microphone were attached to the fiber on both sides, and cork support was used for vibration isolation [12, 13]. The size of the membrane was about 100 × 100 mm. The remaining parts except for the sensing part, including the optical passive components, were sealed with sound absorbing material to avoid unwanted noise, and the optical bench was air isolated from the floor.

The experimental setup is depicted in the photograph in Fig. 5. A 1.55-µm laser diode was biased at dc to produce constant optical power, which was split into two through coupler 1: one to three optical sensors and the other to the acousto-optic modulator (AOM) used to avoid baseband noise. The upper power was again split into three through coupler 2 with each one propagating to the sensor. Output was combined through couplers 4-6 with one output (reference signal) from coupler 3 in order to obtain an interfered signal. After this, the interfered signal was detected at the receiver and converted into an electrical signal that was amplified and filtered to extract the frequency components corresponding to the acoustic wave. Finally, the sound wave was monitored with an oscilloscope.

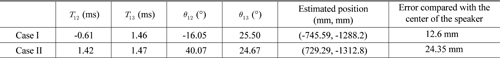
In this experiment, three optical sensors were arranged in a triangle with a horizontal length of 55 cm and a vertical length of 75 cm, as shown in Fig. 6. Position estimation was performed for two cases. In case I, the speaker was placed in front of the array at (−750 mm, −1300 mm) and for case II at (750 mm, −1300 mm). A recorded gunshot sound wave was employed as the sound source.

From the two signals extracted, two different angles θ₁₂ and θ₁₃ were calculated using Eq. (11), and from these, two straight lines were also obtained using Eq. (12). By plotting the two lines, the crossing point corresponding to the position of the speaker was calculated, and finally, the measured value was compared with the actual location of the speaker. Due to a limitation in measurement space, the speaker was placed at a short distance of about 1.5 m from the array and also had a large sound board size of about 100 mm diameter rather than a point source.

Figure 7 shows the gunshot waves detected by the optical sensors in the electrical time domain. The time delay between the sensors was calculated from the arrival times of the waves. In case I, T₁₂ and T₁₃ were −0.61 and 1.46, respectively, and in case II, 1.42 and 1.47, respectively. The estimated positions appeared to be (−745.59 mm, −1288.2 mm) and (729.29 mm, −1312.8 mm), respectively, and compared to the actual position, estimation errors were 12.6 and 24.3 mm, respectively. The results for both cases are summarized in Table 1.

It is likely that these errors were mainly due to the relatively short distance of about 1.5 m between the speaker and the sensor, the assumption of a plane wave, and a large dimension size, i.e. the relatively large size of the speaker and array compared to the distance between them. This could be improved by applying better algorithms for signal processing to estimate the position of arrival, like the minimum variance distortionless response beamformer, a best linear unbiased estimator, or a complex ambiguity function [14-16].

We demonstrated a position estimation method for a sound source using an array of three optical sensors. Each sensor was implemented based on an MZI structure. Especially, to increase the acousto-optic effect, the sensing fiber in one arm of the MZI was surrounded by membranes. The TDOA method was applied to estimate the direction of the sound source. Furthermore, estimation errors appeared to be tens of millimeters compared to the ideal center of the speaker in the presence of practical noises of electromagnetic interference and wall reflection. The proposed method could be used as a good estimation means through a distributed optical sensor network for a sound source placed far away.