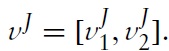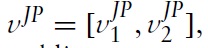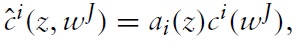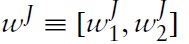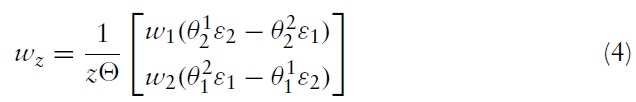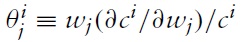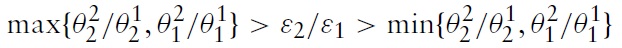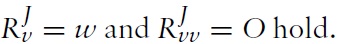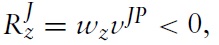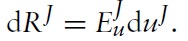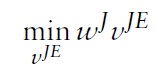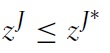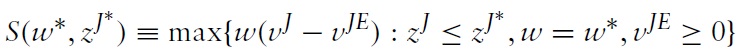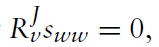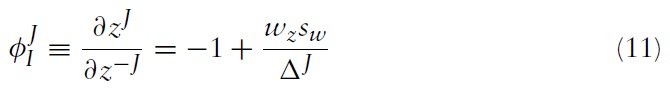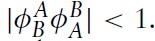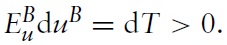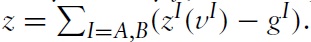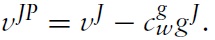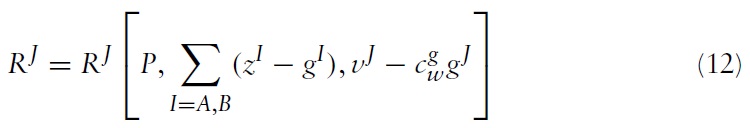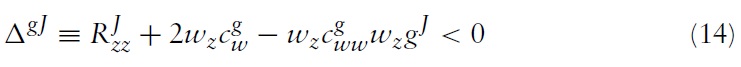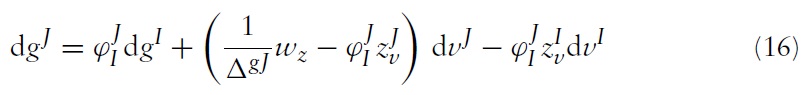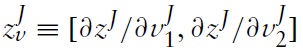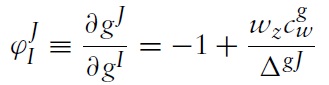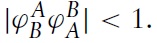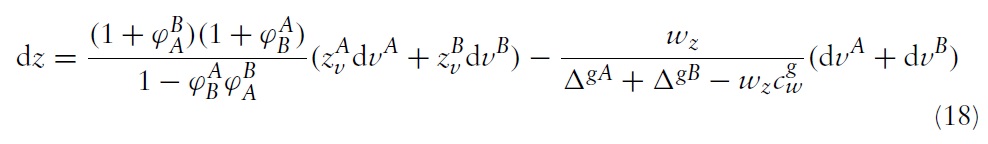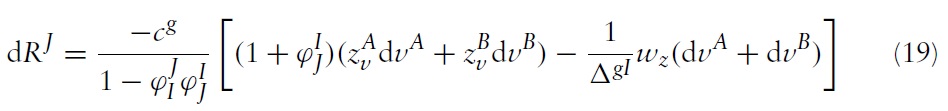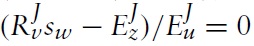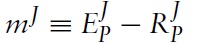Growing concerns regarding global environmental problems, such as climate change and deforestation, have brought attention to the role of financial assistance to developing countries provided by developed countries. Negative externalities arising from such economic activities as production or consumption are often referred to as public bads. Assistance to developing countries is intended to not only improve the welfare of the recipient country, but also reduce international public bads. In this journal, Shibata (2003) presented a rigorous proof for his claim that, in the presence of public bads, thewelfare levels of countries are independent of the pattern of transfers and of the aggregate wealth. This theorem is referred to as Shibata’s second neutrality theorem.1

Shibata (1998, 2003) stresses that most public goods are supplied to mitigate the negative externality arising from such economic activities as production and consumption. The analytical framework employed by Shibata (2003) is a natural extension of the model used in the literature of private provision of public goods (e.g., Warr, 1983; Bergstrom et al., 1986), where it is assumed that each agent can convert one unit of wealth into a fixed amount of private goods, and that the marginal cost of public goods provision is constant.

In the present note,we examine the effects of a change in the factor endowments on welfare by developing a model that consists of two small countries in which two tradable goods are produced using two primary factors of production. In contrast to the claim of the second neutrality theorem, a standard international trade model, such as the Heckscher-Ohlin (H-O) model, states that the determinants of welfare or national income and the determinants of the pattern of trade depend on the distributions of primary factors. Since the second neutrality theorem has significant policy implications for international aid and environmental protection, it is worthwhile reconsidering the theorem in the light of a standard model in the trade theory.2

We demonstrate in this study that the second neutrality theorem may hold in an extended version of the standard model of international trade. However, we also reveal that certain conditions are necessary for the theorem to hold; that is, to mitigate the harmful effects of international public bads each government must have direct control over the resources devoted to production.

The remainder of the present note is organized as follows. In Section 2, we present an extended version of the H-O model developed by Abe (1990) that incorporates the international public bads. Section 3 examines a situation in which the second neutrality theorem is valid. Section 4 shows that the neutrality result does not hold when governments produce a public input to mitigate the damage from international public bads. Section 5 discusses the present results.

1See also Shibata (1998).  2Brakman and van Marrewijk (1998) give a comprehensive reference on the theory of international transfers.Agreat deal of literature has focused on the effects of foreign aid on environmental quality. For example, see Chao and Yu (1999), Hatzipanayotou et al. (2002), Naito (2003) and Takarada (2005).

The analytical framework is based on a small country model of international trade with spill-over public bads, in which two countries, labeled A and B, produce two tradable private goods.3 In each country, there are two fixed primary factors of production, labeled 1 and 2. Factor endowments in country J are denoted by

The primary factors available in the private sector are denoted as

and the vector v^J − v^JP denotes the primary factors used by the public sector.

We consider a situation in which international public bads diminish the efficiencies of the private sectors. The production technology in each private sector, which is assumed to be constant returns to scale in primary inputs, is identical across countries. For given factor prices and a quantity of international public bads, the private sectors minimize their production costs. For analytical simplicity, we specify the unit cost function of the ith private sector in country J = A,B, as

where

and z denote the vector of the factor prices in country J and the amount of international public bads, respectively. The effect of public bads on the unit cost is represented by a_i(z) > 0, where a’_i(z) > 0 and a”_i(z) ≥ 0 are assumed.

Throughout the present note, we shall assume that the factor endowments in both countries satisfy the following assumption.

Let good 1 be a numeraire. If both private goods are produced in country J, the competitive market ensures that:

and

where P denotes a fixed world price of non-numeraire goods. Assuming that the two private industries have different factor intensities without reversal, we can write the factor price vector as the following function of international public bads and the world price:

Since the production technology is assumed to be identical across countries, the unit cost would also be the same. Thus, the factor price equalization theorem is valid, i.e., w^A = w^B holds. Hereafter, in denoting the factor prices, we omit the superscript denoting the country.

Differentiating and manipulating equations (1) and (2), we obtain the effect of international public bads on factor prices, w_z ≡ [∂w1/∂z, ∂w2/∂z], as follows:

where

is factor j’s share in the ith sector, and

4 The sign of Θ reflects the difference in factor intensity between two private sectors. If the ith private sector intensively employs the ith (jth) factor, then Θ becomes positive (negative). The elasticity of the negative effects on the production of the ith sector with respect to public bads is defined as follows:

Equation (4) implies that the price of the production factor that is intensively used in the industry heavily affected by the public bads declines unambiguously. If the international public bads symmetrically damage the production of the two sectors, ε₁(z) = ε₂(z) = ε(z), then equation (4) is simplified as w_z = −(ε/z)w < 0. Accordingly, both factor prices are negatively affected by the public bads. For analytical simplicity, we make the following assumption.

Assumption 2 indicates that for given factor endowments, the private sector’s revenue represented by wv^JP is decreased by an exogenous increase in the international public bads. Based on equation (4), it can be easily verified that if and only if the following inequalities are satisfied, then w_z <0:5

For given values of P, v^JP, and z, the production of private goods in country J can be characterized by the following revenue function:

From the properties of the revenue function,

In addition, the marginal loss caused by the public bads is represented by

where the last inequality follows from Assumption 2.

The revenue function represents a disposable income in the private sector. To focus on the externality affecting the production, we assume that the public bads do not affect the utility of the household directly. In such a situation, a change in welfare is represented by a change in the disposable income. The incomeexpenditure constraint of country J is represented by E^J(P, u^J) − R^J(P, z, v^JP) = 0, where E^J(P, u^J) denotes an expenditure function of country J, and u^J denotes the utility of country J. Since we consider small countries, the change in welfare in country J is given by

The international public bads are generated in both countries. Denoting z^J as the amount of international public bads generated in country J, the total amount of international public bads can be written as z = z^A + z^B. We assume that the amount of international public bads generated in each country depends on the primary factors used in production; that is, the primary factors used in production emit pollution according to a function, z^J(v), as by-products.We have the following assumption on the pollution generating function.

3Although the assumption of small country may appear to be inconsistent with the international public bads, in the present note, we consider a situation where the negative effects caused by public bads spill over to only two countries while each country can trade goods in the global market. In this sense, the public bads considered herein are international but not global.  4To derive equation (4), we use the linear homogeneity of the unit cost functions.  5If we consider the unit cost function that takes the formof ci = (w1/δi) δi [w2/(1 − δi)]1− δi, the condition can be written as max{(1 − δ2)/(1 − δ1), δ2/δ1} > ε2/ε1 > min{(1 − δ2)/(1−δ1), δ2/δ1}.

In this section we consider a situation in which governments in each country can restrict the use of primary factors of production in order to control the quantity of international public bads. It is assumed that the governments purchase at the market price the primary factors, the productive use of which is prohibited.

Thus, the amount of international public bads generated in country J can be written as follows:

where v^JE is the vector of primary factors whose productive use is prohibited by the government. Thus, vJP = v^J − v^JE holds.

Once a target level of public bads in country J, z^J∗, is determined, the government minimizes its cost. The cost minimization problem can be written as follows:

subject to

and

Assuming that an interior solution exists, we obtain the first-order condition for cost minimization as

where μ denotes the shadow price of international public bads. From the first-order conditions, we can define the following function:

The function S(w, z^J) can be interpreted as a restricted revenue function of the private sector for a fixed level of public bads z^J∗. Based on the linear homogeneity of S(w, z^J), we may also write this function as s[w(z, P)]z. Using the properties of a homogeneous function we obtain

and ws_ww = 0. Inserting equation (7) into equation (5), the revenue function becomes as follows:

where z^−J denotes the public bads generated in the country other than J.

So far,we have not explicitly described the budget constraint of the government. It is assumed that the governments levy lump-sum taxes on domestic residents to purchase the factors of production. The budget constraint of the government can then be written as Tax revenue − wv^JE = 0.

Each government maximizes the disposable income described by equation (8) under the Nash conjecture. Noting that

we can write the first-order condition as follows:

In equation (9), the first term of the left-hand side (LHS) represents the marginal damage resulting from the international public bads. The second term of the LHS is the marginal benefit obtained by allowing for additional public bads. The second-order condition, which is assumed to be satisfied, can be written as follows:

Fromthe revenue function given in equation (8), it is clear that the level of public bads is independent of the factor endowments. In addition, the factor prices are independent of the factor endowments. Thus, we have the following proposition.

The intuition behind this result is straightforward. Since the government can have direct control over the primary factors used in production, for a change in the factor endowments, the primary factors available in the private sector, v^JP, do not change as long as the initial level of z^J is optimal in the sense that equation (9) is satisfied.

From the first-order condition, we obtain the optimal response function as φ^J = φ^J(z^−J, P). The slope of the optimal response function is as follows:

Stability around the equilibrium requires

6 As in a typical strategic policy game, equation (11) implies that the optimal level of public bads in one country will change according to the other country’s behavior. Taking into account the presence of a non-contributor, the redistribution of the primary factors between the countries changes the total amount of international public bads, which has been extensively considered by Ihori and Shibata (2006).

When we interpret the second neutrality theorem in the context of foreign aid, the theorem can be considered to focus on the international transfers that take the form of the factors of production. Note that if the transfer takes the form of final goods, the amount of public bads generated in each country does not change. However, the welfare levels will be altered by such a redistribution. For example, let us consider an infinitesimal transfer dT in the formof the final goods from country A to country B. Since the public bads affect production, but not consumption, the welfare effects of the transfer become

and

6Assumption 2 and the second-order condition indicate that although the sign is ambiguous.

A crucial scenario for the second neutrality theorem to hold lies in the policy devices available to the governments. Instead of having a direct control over the primary factors, suppose that the government provides a public input to reduce the public bads.7 The total amount of public bads generated in country J, which is assumed to depend on the factor endowments in each country, is denoted by z^J(v^J). Denoting g^J as the public input provided by country J,we can represent the net amount of international public bads as

Assuming constant returns to scale, the total cost of public input can be written as C^g = c^g(w)g^J, where c^g(w), which is assumed to be identical across the countries, denotes the unit cost function. Thus, the resource constraint in each country becomes

Inserting these expressions into the revenue function we obtain

In this scenario, each government decides the quantity of public inputs to maximize the revenue. Differentiating equation (12) with respect to g^J, we obtain the first-order condition as

The second-order condition can be written as follows:

Fromequation (13), the optimal response of country J can be written as a function of v^J, P, andz^−J − g^−J:

Differentiating equation (15) under the assumption of a small country, we obtain the optimal responses of country J to exogenous changes in g^I, v^A, and v^B.

where

and

denotes the optimal response of country J for an increase in the public inputs provision in country I. Stability around equilibrium requires that

Solving equation (15) for g^A and g^B, we obtain the effects of the change in the factor endowments on the provision of public inputs:

Since dz = dz^A + dz^B −dg^A − dg^B, the net change in the amount of international public bads can be written as follows:

Equation (18) implies that the amount of international public bads at the noncooperative equilibrium can be changed according to the factor endowments.

Moreover, the welfare level may also change. In order to simplify the explanation, we focus on the situation where ε₁(z) = ε₂(z) = ε(z): w_z = −(ε/z)w holds. Taking into account the first-order condition of equation (13), we can denote the change in the welfare as dR^J = −c^g(dz + dg^J) + w dv^J. Using equations (17) and (18), we obtain the change in the disposable income of each country as follows:

From equations (18) and (19), we obtain the following proposition.

In equation (19), if we consider a pure redistribution such that dv^A + dv^B = 0 holds, the second term in parenthesis of the RHS in equation (19) disappears. However, since the term

is not zero, except for the case where

a pure redistribution affects welfare. This means that Warr’s neutrality theorem does not hold.8

7In the literature, Hatzipanayotou et al. (2002) considered a situationwhere the governments supply public goods as a pollution abatement activity.  8Apart fromthe present setting, if the public bad generated in each country is fixed,Warr’s neutrality theorem, which states that the total provision of public inputs is independent of the distribution of primary factors, holds. However, in order for the theorem to hold, the factor prices must be equalized through international trade, which ensures that the marginal effects of public bads on the factor prices are equalized across countries. In this sense, unlike Shibata’s theorem, international trade plays a key role in Warr’s theorem.

We have considered Shibata’s second neutrality theorem in the context of an international trade model. The results presented herein indicate that the theorem is valid as long as the factor endowments available in the public sector are directly determined by the government.

In addition, several assumptions made for the sake of simplicity in Section 3 can be relaxed without changing the results. First, ifwe consider a situationwhere international public bads directly affect the utility of consumers, the expenditure function and the revenue function are given by E^J(P, z, uJ) and R^J(P, s_wz^J), respectively. Thus, the first-order condition can be rewritten as

with

which implies that the neutrality result holds.

Second, the assumption of small countries can be relaxed without changing the results. Let us consider an economy that consists of two large countries. According to Long and Shimomura (2007), if the countries take into account the effect of their contributions of public goods on the world price, the firstorder condition will be

where

denotes the net demand of the non-numeraire goods. In this situation, the firstorder condition is independent of factor endowments.Thus, the second neutrality theorem will hold.

On the other hand, Proposition 2 implies that Shibata’s second neutrality theorem may not hold when we consider a situation where the governments cannot have direct control over the use of primary factors of production. In the real world, governments often directly restrict their productive use of primary factors by means such as land-use regulation, to protect the environmental quality. However, in order for the second neutrality theorem to hold, all primary factors of production generating public bads should be controlled by the government. In addition, the theorem will not hold if one of the two countries adopts the provision of public input as a strategy. In this sense, the non-neutrality result may be plausible.