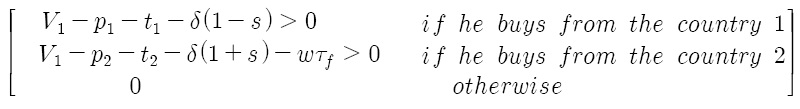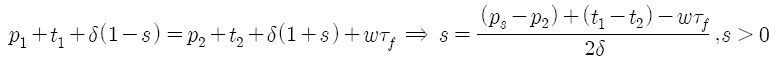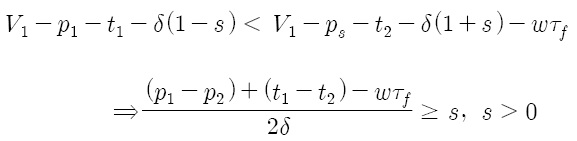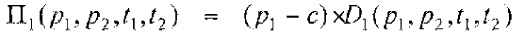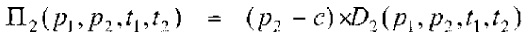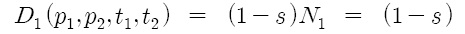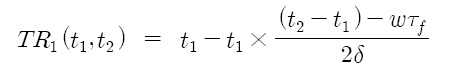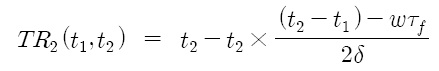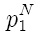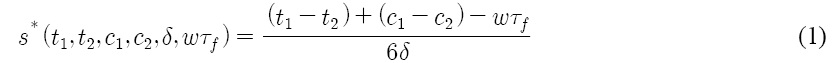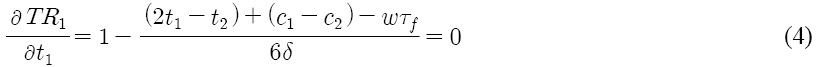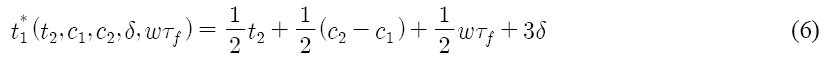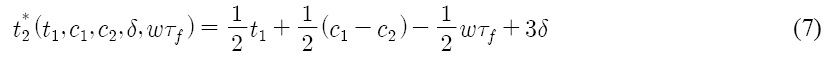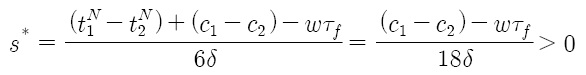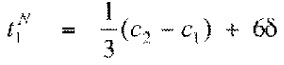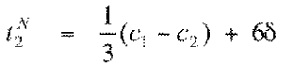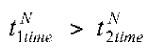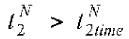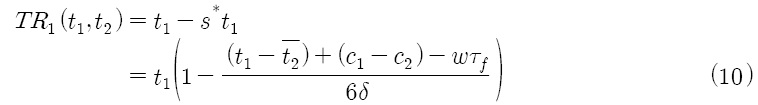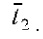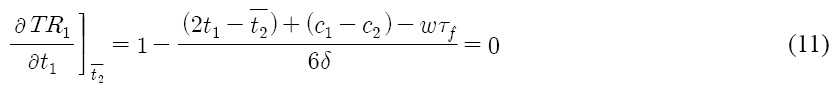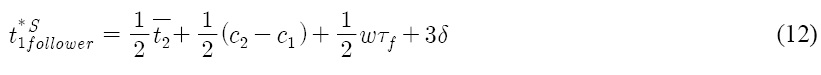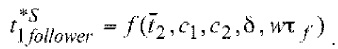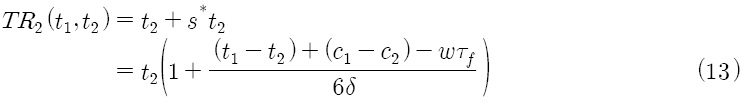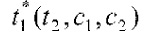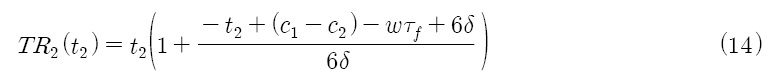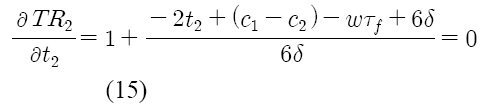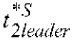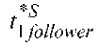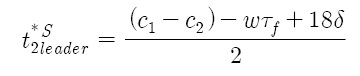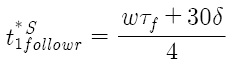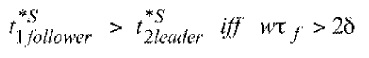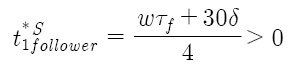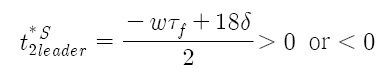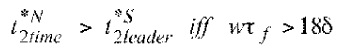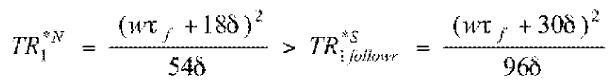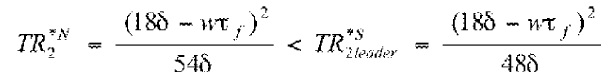When we are looking at ways in which tax policies are made, there are two important factors to consider. First of all, tax regimes between countries are beginning to resemble each other either through tax competition or tax harmonization. In particular, driven on the growth in cross-border transactions and multinational operations, governments are increasingly finding it necessary to incorporate many common elements within their tax policies. Thus, countries are under pressure not to be out of line with their competitors. Secondly, countries are concentrating more and more on maximizing the tax revenue in their own jurisdictions. Faced with domestic opposition to tax rises, governments are focusing on cross-border shopping in an attempt to increase tax revenue.

Tax competition in the taxation of goods and services is an important concern of the theoretical literature on the international tax policy. One of its themes is cross-border shopping which is seen as unavoidable: that is, the purchasing activities of goods and services in one country by consumers of another who try to take advantage of price or tax differentials. This is a phenomenon which can be expected to increase as a consequence of the removal of borders between countries. International goods market integration which enables consumers in one country to consume in other countries inevitably brings up the issue of tax competition between governments. Each country, for example, might be tempted to try to attract cross-border shoppers and hence to gain extra tax revenues by reducing the rate of the commodity tax they levy1.

The logic behind the tax competition is that by slightly undercutting the foreign country’s tax rate, the home government tries to induce the foreign consumers who are engaging in cross-border shopping. A government benefits from undercutting as long as its tax rate is lower than that of the opponent government. International tax competition theory suggests that tax rates will tend to reduce through the process of tax competition in taxes between countries, to induce cross-border shopping competitively. This tends to generate an inefficient outcome in which tax competition results in low tax rates.

Although there has been a growing literature in the area of tax competition which involves not only inter-jurisdictional but also inter-governmental context2, most research on tax competition models examine a noncooperative Nash equilibrium in a tax-setting game. For example, Mintz and Tulkens(1986), Zodrow and Mieszkowski(1986), Wildasin(1988), Lockwood(1993), Kanbur and Keen(1993) examine simultaneous moving tax-setting games.

However, most literature do not explicitly consider the impact of the sequential move on deciding tax rates. For example, in a real-world tax setting, the large region moves first and the small region moves second. This is the most plausible case in the European context. Due to the presence of crossborder shopping, Stackelberg-type sequential move tax setting may turn out to be incentive compatible, while Wang (1999) investigates sequential move tax-setting game regarding tax competition. In our case, the lower-tax country (leader) chooses its own tax rate to maximize its tax revenue while the higher-tax country (follower) will observe the leader’s tax rate before selecting its own tax rate to maximize its tax revenue.

This paper differs greatly from the existing literature in the following two respects. First, we explicitly incorporate the time cost factor engaging in cross-border shopping. The potential for cross-border shopping exists between any two countries where the difference in retail prices or excise taxes of a similar product is sufficiently large enough to offset the transport and time opportunity costs of purchasing the goods in the lower priced foreign markets. This can occur for almost any product but it is usually products which attract excise taxes which demonstrate the widest differentials. Buying goods abroad entails a real cost in terms of leisure foregone, travel and transportation costs. Moreover, the time cost involved in foreign shopping must be included to evaluate the true resources costs.

Cross-border shopping, which is induced by tax rate differentials, involves a waste of resources regarding the transport and time costs involved. Time costs together with travel costs appeared to have major influences on the amount and frequency of cross-border shopping. For example, the purchases of alcoholic drinks (beer, spirits and wine) in France by British consumers crossing the border in the EU context involve resources costs, such as travel and transportation costs. However, the true cost must include the ‘opportunity cost of time’ as well. Without taking into consideration explicitly the time cost factor involved in making the trip, the analysis will probably understate the true resource costs incurred in cross-border shopping.

Time spent in buying a commodity in the cheaper foreign market to cross the border may be an important determinant of the decision for engaging in cross-border shopping activity. In a sense, for some consumers, time is more important than money as the constraining element in consumer’s cross-border shopping activity. Nevertheless, the potential importance of the time cost element has hardly been discussed in the existing literature dealing with the cross-border shopping and tax competition. Moreover, the problem of explicitly incorporating the time cost element into the consumer’s choice engaging in cross-border shopping has never been addressed.

Thus, we explicitly introduce the travel or shopping time required to take part in cross-border shopping activities. Travel time may be a direct commodity or an indirect input for a given purchase or consumption activity. The valuation of time spent in travelling or shopping is important in that the time used is either the opportunity cost of time or the value of time savings. This paper will consider some specific issues in cross-border shopping: the appropriate measure of the value of time as a cost of participating in cross-border shopping activity, and the equilibrium condition for cross-border shopping by taking into consideration the time cost. More specifically, we will examine the effect of time cost on the tax competition.

Secondly, we characterize the Stackelberg equilibrium in tax competition. Tax competition means the process of lowering tax rates and can be identified with noncooperative behaviour with respect to tax rates between governments. Tax competition between governments in the international context can occur when each government attempts to induce cross-border shopping by lowering tax rates at which commodities are taxed. Tax competition is modelled as a non-cooperative game between the two governments with respect to tax rates which are strategic variables in order to maximize tax revenues, bearing in mind cross-border shopping. The tax equilibrium concept utilized is the Nash equilibrium and Stackelberg equilibrium. On the one hand, the Nash equilibrium with tax competition means a situation where each government is choosing its tax rate non-cooperatively and simultaneously, given the tax rates chosen by the other country. On the other hand, in the Stackelberg equilibrium, tax rates are chosen in a sequential manner. That is, the leader-country  sets its tax rate first and then the follower-country chooses its tax rates after observing the leader’s tax rate.

This research addresses strategic aspects of the process of tax competition and places special emphasis on the Stackelberg tax game. This issue has attracted little attention as part of the debate over the process of tax competition. Thus, this paper aims not only to contribute to the literature on strategic tax policy which includes the opportunity cost of time engaging in crossborder shopping, but also to contribute to the tax competition literature by assuming the Stackelberg game. We attempt to investigate the Stackelberg equilibrium in the presence of cross-border shopping and then to compare the Stackelberg equilibrium with the Nash equilibrium.

The paper is organized as follows. Section 2 describes literature surveys. In section 3, we explain the basic model used in the analysis and the main assumptions associated with the model. We consider a linear market consisting of the two countries, two firms and a continuum of consumers who are exposed to cross-border shopping and use a spatial model to analyse the process of tax competition. With the cross-border shopping by consumers, each government attempts to maximize its own tax revenue non-cooperatively so as to attract cross-border shoppers by slightly undercutting the competitor’s tax rate.

In section 4, we analyze the process of tax competition between governments. First, we model a Nash non-cooperative game with respect to taxes which are used as strategic variables, and characterize the Nash equilibrium in tax rates. In section 5, we examine a Stackelberg game. Then, we compare tax rates between the Nash equilibrium and the Stackelberg equilibrium. Finally, section 6 presents concluding remarks.

1In other words, firms and governments are faced with “consumers voting with their cheque books”. In this sense, tax competition is similar to Tiebout’s idea of voting with consumer’s feet.  2The problem of tax competition among jurisdictions or countries has been the subject of a number of major contributions. See, for example, Wilson (1986, 1991), Wildasin (1988, 1991), Zodrow and Mieszkowski (1986), Mintz and Tulkens (1986), de Crombrugghe and Tulkens (1990), Bucovetsky (1991), Chrisiansen (1994), Christiansen, Hagen and Sandmo (1994), Janeba (1994, 1995), Janeba and Peters (1995) and Kanbur and Keen (1993), etc.

It is worthwhile to explore the issue of tax competition in a strategic interaction context, where the choice of tax rates is influenced by the other country’s behaviour and cross-border shopping activities. That is, in an interdependent and integrated world economy, a government’s ability to pursue an autonomous tax policy is restricted not only by the free mobility of consumers, or cross-border shopping, but also the strategic response of the other government. Cross-border shopping by consumers who try to take advantage of tax differentials creates interactions, strategic responses, among countries especially in the European Union. In the presence of cross-border shopping by consumers, each government uses tax policies strategically to manipulate the cross-border shopping in order to try to attract foreign consumers. These foreign consumers attempt to engage in cross-border shopping by taking advantage of tax differentials and to increase additional tax revenues by lowering tax rates competitively. This corresponds to the basic idea of tax competition which is the process of lowering tax rates to induce cross-border shopping. Thus, taxing the consumption of cross-border shoppers might be a significant tool for raising revenue3.

Tax competition with respect to commodity taxes, in particular, in the presence of cross-border shopping has had little attention4 before 1997 when the border controls were removed completely in EU region. Only a few papers have drawn attention to tax competition with cross-border shopping between neighbouring countries with different prices or taxes. Theoretical models of the processes of tax competition based on cross-border shopping have been set out by Mintz and Tulkens (1986), de Crombrugghe and Tulkens (1990), Lockwood (1991) and Kanbur and Keen (1993), Christiansen (1994), Christiansen, Hagen and Sandmo (1994) and Lovely (1994), etc. First of all, the strategically non-cooperative use of commodity taxation in integrated open economies exposed to cross-border shopping has been analysed in the seminal paper by Kanbur and Keen (1993).

There are two driving forces behind the tax competition: one is the mobility of firms and the other is the mobility of consumers, or cross-border shopping. We have two distinctive contrasts to the existing literature. On the  one hand, it is in contrast to the tax competition models of Janeba (1994) which assume that the firms’ location of production is mobile5. In our model, we assume that the firms’ location is fixed at endpoints and instead, consider that the consumers’ location is perfectly mobile in terms of cross-border shopping. The assumption of consumers’ free mobility is in accordance with the tax competition model envisioned by Kanbur and Keen (1993). On the other hand, it is in contrast to Kanbur and Keen’s tax competition model which assumed perfect competition. They assumed that the producer prices of the commodity are both constant and the same between the two countries, but ignored the firms’ location. In general, the underlying models in the tax competition literature are one of perfect competition in the goods market6. In contrast to Kanbur and Keen and the underlying models, we assume imperfect competition in a non-cooperative tax competition game and consider the firms’ fixed location. We will deal with imperfect competition in the sense that case of imperfect competition may be of particular interest in the context of cross-border shopping since imperfect competition may be a reason why prices differ and why people want to shop abroad7. The researchers will also investigate price as the relevant strategic variable in a duopolistic competition and apply it to study price competition among firms.

The main theme of this paper is in same spirit of Kanbur and Keen’s model8 which analyzes commodity tax competition in the presence of cross-border shopping with finite transportation costs. Like Kanbur and Keen’s model, the basic idea of the tax competition is based on the free mobility of the consumers expressed in terms of cross-border shopping induced by tax differentials in combination with non-discrimination or origin tax principle on the consumption by foreign consumers. In our model, the assumption of consumers’ mobility is the main driving force behind tax competition in the sense that consumers’ mobility is the result of difference in prices of firms and in taxes of governments.

However, we are significantly different from Kanbur and Keen (1993) and Lee (2008, 2010) in two respects. First, we introduce more general cross-border shopping structure by incorporating the opportunity cost of time in addition to transportation costs. Second, we employ the Stackelberg equilibrium concept so as to examine tax competition.

3This is in contrast to the existing tax competition literature in the sense that it has been recognized that taxing mobile factors like capital might not be a tool for that it has been recognized that taxing mobile factors like capital might not be a tool for raising revenue.  4On the contrary, the phenomenon of tax competition with respect to capital taxes in the inter-jurisdictional context has received much attention from local public finance specialists. See, for instance, Wilson (1986, 1987), Zodrow and Mieszkowski (1986), Wildasin (1988, 1991), Hoyt (1991), Bucovetsky (1991), Janeba (1994, 1995) , Janeba and Peters (1995), etc.  5Wilson (1986, 1987), Zodrow and Mieszkowski (1986), Wildasin(1988, 1991), Hoyt(1991), de Crombrugghe and Tulkens (1990), Bucovetsky (1991), Janeba (1994, 1995), Janeba and Peters (1995) assumed the mobility of firms or capital. This research has assumed that firms respond to tax differentials and change their locations.  6See, for instance, Kanbur and Keen (1993), Mintz and Tulkens (1986), Wildasin (1988), Janeba (1995), Christiansen, Hagen and Sandmo (1994), etc.  7Christiansen (1994) analysed optimal commodity taxation for an economy exposed to cross-border shopping under perfect and imperfect competition and derive optimal rules for commodity tax.  8Kanbur and Keen’s tax competition model is unique in that with not only considering cross-border shopping by consumers and finite transportation cost explicitly in an integrated economy, but also assuming perfect competition and different country size, the two governments will use tax rates on the commodity consumption as strategic variables in a Nash non-cooperative game and compete in tax rates, thereby maximizing their own tax revenues non-coopera tively and independently.

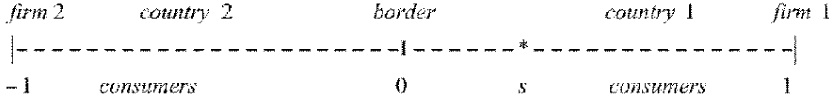
Subsequently, we use a duopolistic spatial model to develop a model of price and tax competition. We consider a linear market consisting of the two countries, two firms and a continuum of consumers who can engage in crossborder shopping. On a line market of length 2, two firms produce a single homogeneous product with constant marginal costs and supply the goods for the consumers at home or abroad. Two firms are located, respectively, at the endpoints of this line segment: that is, the location of the two firms is fixed. Each firm competes in price as strategic variable and maximizes its own profit independently. Consumers are evenly distributed along the linear market and buy one unit of the commodity per unit of time. Since the product is homogeneous, consumers will buy the commodity from the firm that quotes the lowest delivered price. Transportation costs are paid by consumers and are assumed to be a linear function of distance10. In addition, consumers must pay time cost incurring from the foreign shopping.

Two governments might be tempted to try to attract cross-border shoppers and hence to gain additional revenues. Both governments impose taxes both on domestic consumption and cross-border purchases at a uniform rate. Each government competes in tax rate as a strategic variable and maximizes its own tax revenue non-cooperatively. Two firms and two governments behave in a non-cooperative fashion. The equilibrium concept we use is the sub-game perfect equilibrium of this two-stage game.

To present the assumptions described briefly above, we will assume them more formally way as follows.

The geographic market consists of the two countries, two firms and a continuum of consumers, and is represented by the interval [-1, 1].

The following Figure illustrates these assumptions.

Consumers have an incentive to engage in cross-border shopping by total price differentials so as to take advantage of price or tax differentials.

Each economic activity would take time, however, thus preventing the consumer from engaging in some other activity during that period. In making choices of how to spend time in engaging in cross-border shopping, consumers would have to take into consideration not only the monetary cost of cross-border shopping activity such as purchasing cost, transportation cost and travel costs, but also the opportunity cost of the time the cross-border shopping activity entails.

We consider explicitly that cross-border shopping entails the time cost involved in the context of ‘generalized’ travel cost method. Conventional travel cost method considers only travel costs, and treats the distance from the home to the shopping destination and the cost per mile travelled as exogenously given. However, there is a general agreement that the opportunity cost of time spent travelling should be counted among the costs of travel. Assuming that travelling is costly and the transport cost, δ, increases with distance, s, then it follows that the visitation rate or shopping trip diminishes as the cost of visiting or travelling increases. Thus, we define the function Φ to be assumed to be finite and linear function of distance12:

where s is a traveling distance that consumers must travel to reach the firms or shops from which he buys.

We take explicitly into consideration the travel cost and time cost of travel. It will be desirable that the total costs of cross-border shopping activity involved be decomposable into its money and time components. If we take the total cost of a trip to be given by the sum of the money cost and the money equivalent of the time cost, then the generalized travel cost of travelling from the home country to the foreign shopping country is given by:

where s is the travel distance (in miles) from home to abroad, δ is the transportation cost (in pounds) per unit distance of automobile travel which can be taken to be equal to a constant travel cost per mile, such as £1 permile, τ_f is the travel time (in hours or minutes) from home to foreign market, and w is the value of travel time which may be, for instance, taken to be equal to one-third the average wage rate.

This generalized travel costs method can be used for empirical studies. The obvious problem of including travel time valuations explicitly is that time consumption has no market value. That is, whereas the variable cost of automobile travel may be reasonably estimated from market prices for gasoline, oil, etc., the valuation placed on travel time is highly subjective, varying from individual to individual and from transport mode to transport mode. Attempts can be made to empirically include the cost of time into the traditional travel cost method. For example, the estimates of the cost of travel time may range from maximum to average or to minimum wage rate per hour.

Since time is a limited resource that can be saved and employed elsewhere, it has an opportunity cost or scarcity value for each individual. If time is discretionary free time and its alternative use is for work, then its opportunity cost is the wage rate. However, because of labour contracts and other constraints which may limit flexibility in the use of time, time allocation may be pre-committed and thus the trade-off may be between time for travel and time for leisure activities (e.g. rest, sleep, sports, etc.). In this context, the opportunity cost of travel time would be the value placed on alternative uses of leisure time by individuals. For that reason, the opportunity cost of travel time may differ from the wage rate. In this context, a rationale is needed to explain why the value of travel time is, in general, less than the wage rate. If time is valued at its opportunity cost minus its commodity value, then the discussion may indicate two possible reasons. First, the value of travel time may be less than the wage rate because travelling is enjoyable and has a positive commodity value. Second, the value of travel time may be less than the wage rate because of constraints which prevent the substitution of travel time for alternative uses of time.

Now, we formally analyze the choice of cross-border shopping by consumers under the inclusion of time cost factor. Suppose, for instance, a consumer is located at the distance of s（s＞0）from the border. Then, in addition to paying the gross prices inclusive of tax for the good purchased, the consumer must bear both the linear transportation costs per unit of distance and the opportunity cost of time as follows:

Denoting prices by P₁ and P₂, taxes by t₁ and t₂ , transportation cost by δ, and time opportunity cost by wτ_f, then the total cost to a consumer of country 1 for domestic purchases is p₁＋t₁＋δ（1－s）. The total cost of a consumer of country 1 to purchase abroad, on the other hand, is p₂＋t₂＋δ（1＋s）＋wτ_f In other words, the total price of going to the two firms for a consumer located at s is as follows:

Under these assumptions, consider, without loss of generality, the decision problem of a consumer in the country 1. Consumer’s surplus enjoyed by consuming the goods at home or abroad must be greater than zero. If the consumer’s reservation price of the country 1 is denoted by V₁ , then the surplus of a consumer at s(s＞0) is expressed as follows:

Now, a consumer concerned will be indifferent between buying from the firm 1 in country 1 and buying from the firm 2 in country 2 if the following condition is satisfied:

where s represents so-called ‘critical or indifferent distance’ at which consumers are indifferent between purchasing in country 1 or in country 2.

Cross-border shopping may occur by the comparison of consumer’s surplus at home and abroad. A consumer concerned will engage in cross-border shopping towards the foreign country, if and only if, the consumer’s surplus gained by cross-border shopping abroad exceeds that from buying at home. This can be represented as follows:

That is, this is referred to the cross-border shopping condition for a consumer located at s＞0. This is different from Kanbur and Keen (1993) and Lee (2008, 2010).

Note that cross-border shopping can occur by the price difference and time costs as well. This is in contrast to Kanbur and Keen in the sense that under the assumption of imperfect competition, cross-border shopping is affected not only by the tax differentials but also the price differences. Furthermore, this is also juxtaposed to Lee (2008, 2010) in the sense that it contains the time opportunity costs.

On the supply side, assuming fixed locations, each firm chooses its profit-maximizing price taking as given both the price charged by the other firm and the taxes imposed by the two governments.

Thus, the payoff functions are given by the profit functions of the two firms:

where D₁ and D₂ are demand functions.

The demand functions, denoted by D₁ and D₂, of each firm can be derived by the consumption of consumers, that is, by both the consumer’s location and the number of consumers. Assuming s≥0 and N₁＝N₂＝1, the demand functions are simplified as:

where s denotes the location of the consumer who is indifferent between firm 1 and firm 2, and N_i,i＝1, 2, indicates the number of consumers.

This is a non-cooperative two-player game in which players are two firms, strategies are prices of each firm and payoffs are profits to the two firms, given tax rates set by governments. Therefore, the concept of price equilibrium is the Nash equilibrium of non-cooperative game.

We assume that each government is behaved as Leviathan13 who maximizes his own tax revenue14. Each government raises his tax revenue through the commodity taxation which is imposed uniformly on the consumption purchased by home consumers or foreign consumers15.

The following assumptions make the tax competition idea as strong as possible.

Therefore, with the extent of the cross-border shopping as s＝［（t₂－t₁）－wτ_f］/2δ, we can derive the two governments’ payoff functions, or tax revenue functions. Assuming that symmetric country size and t₁＞t₂ for analytical conveniences, the revenue functions are then simplified as follows:

The last assumption relates to the timing of the game and the equilibrium concept. We will consider the two-stage non-cooperative game: price competition in the second stage and tax competition game in the first stage. The timing of the game is as follows.

The equilibrium concept we use is sub-game perfect Nash equilibrium in stage 2. We assume that firms choose prices after taxes have been set. The price and tax strategies are assumed to be played one at a time in a twostage process. The division into two stages is motivated by the fact that the choice about taxes is prior to the decision on prices. Governments anticipate the firm’s price decision and consumer’s consumption decision and set tax rates strategically. Thus, taxes are chosen by the governments in the first stage and then prices by the firms in the second stage. However, tax rates are chosen simultaneously or sequentially. That is, we characterize the Nash equilibrium and Stackelberg equilibrium in separate way. First, we examine the Nash equilibrium tax rates and then derive the Stackelberg equilibrium in tax rates. Finally, we compare both equilibria.

9This section is based onLee (2008, 2010).  10This model is analogous with the usual Hotelling-type duopolistic spatial models. See Hotelling (1929).  11For the case of asymmetric country size, see Kanbur and Keen (1993), Wilson (1987), Wildasin (1988), Bucovetsky (1991), Janeba and Peters (1995), etc.  12On the contrary, Christiansen, Hagen and Sandmo (1994) considered ‘free’ cross border shopping: that is, consumers can move cost free across the border to make their purchases abroad. In this case, they pay the price inclusive of tax charged abroad. However, our model like Kanbur and Keen assumes costly or finite cross-border shopping.  13For the problem of the Leviathan model in tax competition, see McLure(1986). For instance, Kanbur and Keen(1993) dealt with the model of tax revenue maximization as government objective.  14Most of the tax competition literatures assume that each government maximizes national welfare or consumer’s surplus. In that case, tax competition works as follows: each government designs its tax policy so as to maximize the welfare of its representative resident. In carrying out the maximization problem, the government must take into account the resource constraints, and also takes as given tax rates employed by the other governments.  15For the welfare effect of cross-border shopping, refer to Trandel (1992) and Lovely (1994). Trandel used a spatial model of competition to analyse the welfare effect of border crossing by consumers. Lovely developed a model of consumer border crossing induced by commodity tax differentials and examines the welfare effect of commodity tax evasion by engaging in the cross-border shopping.  16That is, the two governments do not discriminate between home consumers and foreign consumers who are engaged in cross-border shopping towards home country.  17For an economic discussion of the destination and origin principles, see Sinn(1990), Keen and Lahiri(1998), Lockwood(1993), etc.

This is a two-stage non-cooperative game in which two firms compete competitively in prices in the second stage, and then two governments compete non-cooperatively in taxes in the first stage. For the result of price competition in the second stage, refer to Lee (2008).

Now, we consider solving the first stage in which each government chooses tax rate in a non-cooperative manner so as to maximize its own tax revenue. Substituting

and

into marginal consumer s, we can find s^* as a function of tax rates, marginal production cost, transportation and time costs:

where s^* represents the extent of cross-border shopping induced by the tax and cost differentials and transportation and time costs.

Using this condition, we can obtain the tax revenue functions of the two countries as follows:

These two revenue functions, which involve the tax differential of the two countries, the cost difference of the two firms and the transportation and time costs by which the cross-border shopping is induced, imply that even though the two countries do not explicitly coordinate their tax policies between them, each country must take into account the tax system of the other in designing its own tax policy while keeping in mind the cross-border shopping. Given whatever tax rate its competitor is imposing, therefore, the existence of cross-border shopping may provide each government with an increased incentive to cut its own tax rate. The intuition is that when cross-border shopping induced by tax differentials encourages consumers to purchase in lower tax country, there will be increased competition between two governments in order to attract cross-border consumers into their own country and thereby to increase extra tax revenues.

In the Nash non-cooperative-tax-competition game, each country seeks to maximize its own tax revenue independently with respect to its own tax rate, taking the tax rate of the other government as given. For instance, the country 1 chooses its own tax setting, t₁, in order to maximize its own objective tax revenue function, TR₁, taking foreign tax rate, t₂, as given.

Mathematically, the first-order condition for equilibrium in the first stage when choosing tax rates independently and non-cooperatively may be written:

For instance, each country should choose its tax rate independently in order to maximize its own tax revenue in such a way that no perturbation of its tax policy could raise its tax revenue. Thus, the equilibrium tax rates are given as:

These two functions are called the reaction, or best response, functions for two governments, respectively.

Proof: The Nash non-cooperative equilibrium in taxes is given by the intersection of the two reaction functions. Algebraically, it may be found by solving the equilibrium taxes simultaneously.

The Nash equilibrium taxes in both countries depend both on the cost differences between two firms and on the transportation and time costs, and resulting in an asymmetric equilibrium.

Corollary 1: Under the assumption of asymmetric costs, the extent of cross-border shopping, s^*, in the Nash equilibrium depends on both the transportation cost and time opportunity cost in addition to the cost difference:

This implies that assuming asymmetric production costs, both an increase in transport costs and an increase in the opportunity cost of time will reduce the extent of cross-border shopping. Thus, cross-border shopping is influenced by both transportation and time opportunity costs incurred by consumers.

We first compare the Nash equilibrium under the time cost consideration between two countries and then compare the Nash equilibrium without time cost and the Nash equilibrium with time cost. From the equations (8) and (9), the sub-game perfect Nash equilibrium in tax rates without consideration of time costs factor is given as18:

Corollary 2: Assuming the same production cost and comparing Nash equilibrium under the consideration of time cost factor, the country 1 has higher tax rate than the country 2:

18For this derivation, refer to Lee (2008, 2010).

In this section, we turn to apply the Stackelberg model game to the tax competition. In its broadest sense, the tax competition describes a process in which governments set their tax rates non-cooperatively so as to maximize their own country’s tax revenues, given the tax rate pursued by other governments. Tax competition could arise in such situations that each country might be tempted to try to attract cross-border shoppers. Hence, gaining extra revenue by lowering the rate of commodity taxes they levy in the hope that it would induce a sufficiently large increase in tax base to compensate for reduction in the tax paid on each unit of the cross-border shopping.

In this section, we use a Stackelberg game to analyze the tax competition more realistically. One government makes a choice of tax rate before the other government. The Stackelberg model is often used to describe industries in which there is a dominant firm, or a natural leader. For example, IBM is often considered to be a dominant firm in the computer industry. Similarly, we may consider the lower tax country to be a Stackelberg leader in the sense that its tax rates serve to induce cross-border consumers toward its country. Usually, the higher tax country will wait for the announcement of the lower tax country and then adjust its own tax decision accordingly. Therefore, we attempt to model the lower tax country playing the role of a Stackelberg leader and the other higher tax country being a Stackelberg follower.

The logic behind the tax competition is as follows. By lowering tax rates, each government tries to induce foreign consumers into its jurisdiction. Each government is attempting to attract foreign consumers by lowering tax rates. Thus, it is shown that the best response to tax competition is to lower its tax rates against opponents non-cooperatively. Since both governments have the same incentive, the result is an unrestricted tax competition.

We assume that the lower-tax country 2 is the Stackelberg leader which sets its tax rate,t₂. The higher-tax country 1 is the Stackelberg follower, which responds by setting its tax rate, t₁, after observing the leader’s tax  rate. Then, what tax rate should the leader choose to maximize its tax revenue? The answer depends on how the leader thinks that the follower will react to its choice. Presumably the leader should expect that the follower will attempt to maximize tax revenue as well, given the choice made by the leader. The equilibrium concept we employ is the backward induction. We adopt a two-stage sequential approach: in step 1, we solve the follower country’s reaction function and in step 2, we solve the leader country’s reaction function.

First, we solve the follower country 1’s problem as follows. The tax revenue of country 1 is given as:

The follower’s tax revenue depends on the tax rate of the leader, but from the viewpoint of the follower the leader’s tax rate is predetermined,

The follower chooses its tax rate to maximize its tax revenue:

Then, the optimal tax rate of the follower country in a Stackelberg game is derived as:

where the superscript S denotes the Stackelberg game. This implies that the revenue-maximizing tax rate of the follower depends on the tax rate chosen by the leader, cost difference, transportation and time costs:

Second, we solve the leader country 2’s problem. The tax revenue of country 2, or the leader is given as:

The leader is aware that its action influences the tax-rate choice of the follower. Hence, when making its tax-rate choice it should recognize the influence that it exerts on the follower. Now, substituting

for t₁ in equation (13) and arranging it yields:

The leader chooses its tax rate to maximize its tax revenue, taking into account the follower’s reaction function, as follows:

Then, the leader country’s optimal tax rate is derived as:

Finally, substituting

for t₂ in

gives the optimal tax rate of the follower as:

Thus, the following propositions characterize the Stackelberg equilibrium.

This implies that the optimal tax rate in a Stackelberg game depends on the cost difference, transportation cost and the opportunity cost of time.

Corollary 3: Assuming that production costs of two firms are the same, c₁＝c₂, then optimal tax rates in a Stackelberg game are given as:

This corollary implies that assuming the same production cost, the optimal tax rate in the Stackelberg game depends on the transportation cost and the opportunity cost of time. In particular, the transportation cost influences positive effect on the tax rates in both countries, while the opportunity cost of time has a different effect on the tax rates of both countries.

Therefore, there is an asymmetric equilibrium in the Stackelberg model. This implies that the leading country can use its tax rate strategically.

Corollary 4: Assuming the same production cost and comparing the Stackelberg equilibrium under the consideration of time cost, country 1 has higher tax rate than country 2, if and only, if the opportunity cost of time is greater than transportation cost:

Corollary 5: Follower, or higher-tax, country does always decrease its tax rate from the increase in cross-border shopping. However, the leader, or lower-tax, country can either decrease further or even increase its tax rate from the increase in cross-border shopping. For the leader, the former implies that the cross-border shopping leads to more competition in tax, while the latter shows that there will be a convergence in tax rates between two countries:

Now, it is of great interest to compare the Stackelberg equilibrium with the Nash equilibrium.

The intuition behind this proposition is straightforward. In the Nash equilibrium, when choosing its tax rate, each country ignores the beneficial beneficial effect19 that raising its tax rate would have on the tax revenue of the other country by increasing cross-border shopping. However, in the Stackelberg equilibrium, the follower country will lower its own tax rate if it observes a leader’s low tax rate. The leader country knows this also and consequently lowers its own tax rate with more concern about the impact on the cross-border shopping. Thus, the Stackelberg tax rates are lower than the Nash tax rates.

Here, we had a natural assumption that the opportunity cost of time is greater than the transportation cost. It makes sense since most of cross-border shoppers engage in occasional or opportunistic shopping while they are traveling. Otherwise, much more consumers would participate in cross-border shopping.

This implies that although the leader, or low-tax, country sets a lower tax rate in the Stackelberg equilibrium, it collects more tax revenue in the Stackelberg equilibrium because of the presence of outward cross-border  shopping.

19The beneficial effect implies that when making its own tax choice non-co-operatively, each government ignores this external effect. The most common approach to analyse the strategic interactions between countries in such a beneficial externality relation is to find first-order cross derivatives of its own tax revenue function with respect to tax rate of the other country: ∂TR1/∂t2＞0 and∂TR2/∂t1＞0. There is a beneficial effect associated with cross-border shopping: that is, taxpaying consumers engaging in cross-border shopping may add surpluses in tax revenue of the other country. Thus, there is an incentive to lower tax rates in order to induce cross-border shopping. To see the external or beneficial tax effect in the Nash non-cooperative tax game, refer to Lee (2010)

In this paper, we have examined international tax competition in the crossborder shopping setting along with assuming imperfect competition. In an interdependent and integrated world economy, a government’s ability to pursue an autonomous tax policy is restricted not only by the free mobility of consumers, or cross-border shopping, but also by the strategic response of the other government20. That is, under the borderless open economy, each government faces a choice between a restriction of the tax rates they can impose through the pressure of competition in a non-cooperative manner (i.e., tax competition) and a constraint by agreement between countries in a cooperative way (i.e., tax harmonization or tax cooperation). In principle, each country which tries to maximize its own tax revenue has an incentive to engage in tax competition by lowering its own tax rate independently in order to induce cross-border consumers.

In particular, in the EU context, tax competition will push tax rates down unless attempts are made at some harmonization of rates at least in neighboring countries. For this reason, the EU attempted to alleviate this problem by asking member countries to adopt ‘minimum tax rates’ for the excise duty21.

We focused on the equilibrium structure of the Stackelberg game in tax setting and then compared it with the Nash equilibrium when we explicitly incorporate the opportunity cost of time. The leader country collects more tax revenue if it adopts the Stackelberg tax-setting model rather than the Nash tax scheme because of the cross-border shopping incorporated the time cost factor.

Some results contribute to the literature in the following aspects. First, assuming the same production cost, the optimal tax rate in the Stackelberg game depends on the transportation cost and the opportunity cost of time. In particular, the transportation cost influences positive effects on the tax rates in both countries, while the opportunity cost of time has different effects on the tax rates of both countries (Proposition 3). Second, follower, or higher-tax, country does always decrease its tax rate from the increase in cross-border shopping. However, the leader, or lower-tax, country can either decrease further or even increase its tax rate due to the increase in cross-border shopping. Especially, in the leader’s case, the former indicates that the cross-border shopping may lead to more competition in tax, while the latter tells us that there will be a convergence in tax rates between two countries without harmonizing efforts (Corollary 5). Third, comparing tax revenues in the Stackelberg equilibrium with those in the Nash equilibrium, the follower country collects higher tax revenue in the Nash equilibrium, while the leader country collects higher tax revenue in the Stackelberg equilibrium (Proposition 5).

Based on the assumption of a more general cross-border shopping structure, the derived Stackelberg equilibrium in tax rates will shed some light on the optimal tax policy design for tax competition and tax harmonization in EU. For example, judging from the Stackelberg tax rates, concerted effort to harmonize member’s tax rates in EU would harm the follower country in terms of tax setting.

It would be of great interest to apply the model we have examined to South Korea, where many Chinese and Japanese cross-border consumers travel to Korea to take advantage of the cheap foreign exchange in Korea. Driving forces behind cross-border shopping in Korea is, at present, cheap foreign exchange rates rather than lower tax rates. However, tax rates will become a main driver in the foreseeable future. This remains for further research.

20According to Razin and Sadka (1994), Nash noncooperative tax competition in an international context arises when each of the competing governments cannot exercise significant market power in setting its tax rates.  21For an analysis on minimum tax rate system in tax competition, see Kanbur and Keen (1993).