Ag(Ta,Nb)O3 materials have a perovskite structure with a low loss tangent. These materials have been widely researched for their applications as high-frequency, passive components. Also, Ag(Ta,Nb)O3 materials have weak frequency dispersion with high dielectric permittivity which gives them enormous potential for use in electronic components, including the filters, and embedded capacitors. Therefore, our research will discuss the structural and electrical relaxation properties of Ag(Ta0.8Nb0.2)O3 ceramics for device applications. We will investigate using X-ray diffraction to understand their structural properties and will analyze voltage dependent leakage current and timedependent relaxation behavior to understand their material properties.
These days, perovskite-type ferroelectric materials have drawn substantial attention due to their properties for dielectric and piezoelectric applications, like for capacitors or infrared sensors [1]. In this paper, we introduce Ag(Ta,Nb)O3 materials which have highly dielectric properties and low dielectric losses. These materials are potentially useful for wireless-communications, microelectronic technologies, and the miniaturization of microwave components [2-4]. Comprehensive study of the dielectric properties of microwave, and sub-millimeter to infrared spectroscopy have proved that Ag(Ta,Nb)O3 are good ferroelectric materials [5].
Ferroelectrics are brilliant materials which have a nonlinear behavior in their dielectric permittivity, with an applied electric field and ferroelectric characteristics strongly dependent on composition, processing condition, microstructure, applied electric field, operating temperature, and frequency. Ag(Ta,Nb) O3 (hereafter ATN) material can be adapted to microwave applications due to their low loss tangent of around 3×10-3 from the 1 kHz to the 100 GHz range [6]. Many studies have been carried out to investigate how to improve the dielectric properties through a conventional mixed method [7-10]. ATN shows an interesting dependence of physical properties, such as phase transition and the dielectric permittivity on the Ta/Nb com-position. According to Kania, perovskite ATN ceramics showed high dielectric permittivity and low loss tangent with temperature-stable dielectric properties [6]. Therefore, ATN can be a useful material for applications in wireless communications, microwave technologies, and miniaturization of components [11]. The electrical properties, including the leakage current and the relaxation behavior, were found to be greatly influenced by the composition. From this point view, we analyze the characteristics of the various compositions of ATN ceramics.
To prepare Ag(Ta,Nb)O3 ceramics, Ag(Ta,Nb)O3 powder was prepared from Ag2O, Ta2O5, and Nb2O5 powders with a high purity of 99.9 % by a conventional mixing method. Ag2O, Ta2O5, and Nb2O5 powders were employed to prepare the stoichiometric composition of Ag(Ta,Nb)O3 ceramics. After being ballmilled for 24 hours with ZrO2 balls and then dried, Ag(Ta,Nb)O3 powders were calcined at 940℃ for 2 hours and then slowly cooled. According to the different Ta / Nb compositions of Ag(Ta,Nb)O3 materials, the sintering temperatures have to be different[12]. The refined powder size was of around 127 μm. After granulation, these powders were pressed at 1 ton/cm2. After pressing, the Ag(Ta,Nb)O3 ceramics were sintered at 1,140℃ for 4 hours.
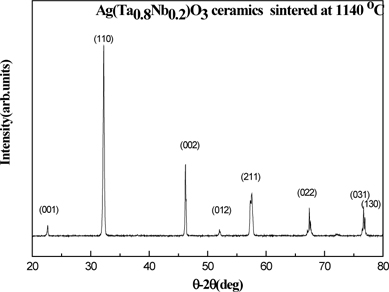
Finally, silver paste was applied to the upper and bottom side of the Ag(Ta,Nb)O3 ceramics. We measured the crystalline structure of the Ag(Ta,Nb)O3 ceramics using X-ray diffraction (XRD) patterns (
Figure 1 displays the
where Ccos
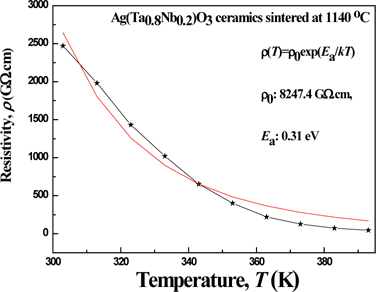
Figure 2 illustrates the temperature dependent resistivity of the Ag(Ta0.8Nb0.2)O3 ceramics. The resistivity of these ceramics was calculated from the resistance by considering the geometry. During calculation, the total electrode area of the vertical-type ceramic capacitor and the length of the current path between the electrodes were considered. Due to the current-voltage properties and their relationship to temperature, we proposed the Arrhenius equation to describe the temperature-dependent resistance behaviour:
where
From Eq. (3), the calculated temperature coefficient of this thick film was -5.08×10-11 K-1. The simulated ρ0 of the Ag(Ta0.8Nb0.2)O3 ceramics was 8,247.4 GΩ.cm, and the calculated activation energies were around 0.31 eV [13].
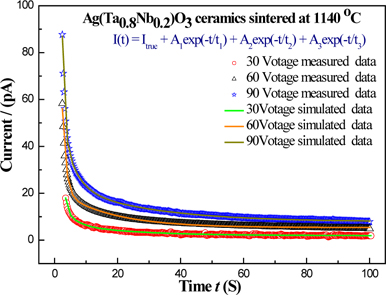
Figure 3 indicates the transient current
Therefore, we defined these time-dependent leakage currents as polarization currents ‘Itransient’ from the different types of dipole motions and the true leakage current ‘Itrue’. From the simulation in Fig. 3, we may claim that the time-dependent current behavior can be expressed by the relaxation of dipole motions in the polarization process [14]:
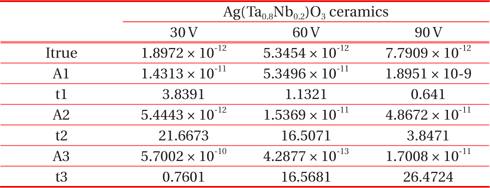
The simulated values are listed in Table 1.
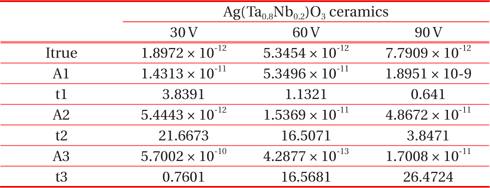
Fitting value of the transient current I recorded in the time domain for Ag(Ta0.8Nb0.2)O3 ceramics.
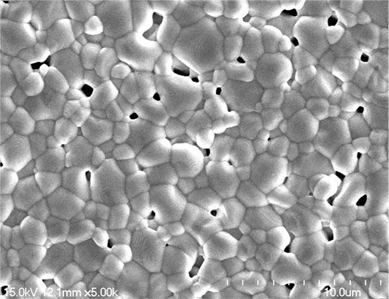
Figure 4 shows the SEM image of the Ag(Ta0.8Nb0.2)O3 ceramics sintered at 1,140℃. We observed the grain shape and size of the Ag(Ta0.8Nb0.2)O3 ceramics, and we can argue that the Ag(Ta0.8Nb0.2)O3 ceramics were well crystallized. Due to the volatility of Ag ions during the sintering process, a small porosity of the Ag(Ta0.8Nb0.2)O3 ceramics was observed. We can conclude that the porosity and grain size in the Ag(Ta0.8Nb0.2)O3 ceramics played a role in increasing the transient current I recorded in the time domain for the Ag(Ta,Nb)O3 ceramics.
In this research, we analyze the structural and electrical characteristics of the Ag(Ta0.8Nb0.2)O3 ceramics. Using X-ray diffraction analysis, we could see that ATN ceramics had a perovskite structure with pseudo-cubic systems. The calculated lattice parameters
We conclude Ag(Ta0.8Nb0.2)O3 ceramics had a well crystallized structure and brilliant electrical properties for application as passive components.