The barbel steed (Hemibarbus labeo Pallas, 1776) is a primary freshwater fish that prefers deep pools with running water in lower and middle river reaches, and forms schools. The species is carnivorous, feeding on shrimps and aquatic insects (Lin et al., 2010). It is well-defined and can be distinguished from congenerics based on the broad and thick lateral lobes of the lower lop with folds (Yue, 1998). It is widely distributed in eastern Asia, from Vietnam to Russia. The limited ability of the species to migrate makes this family of obvious biogeographical interest, because their distribution closely reflects the geographical evolution of the landscape (Durand et al., 2002). This species is popular with anglers, and is a palatable, economical fish that is rich in nutrients (Novomodny et al., 2004).

Studies of H. labeo have been mainly conducted in Asia, almost all in China. Some researchers have used molecular methods to identify Hemibarbus species. Lv (2008) showed that the genetic diversity of H. labeo was high. They also inferred that H. labeo from Korea and Japan should be treated as nonym of H. labeo from China. Artificial reproduction of the species has also been studied recently. Xu et al. (2009) studied the reproduction of reared H. labeo in the Wusuli River. Luo et al. (2011) spent much time studying techniques for the artificial propagation of H. labeo. However, studies on growth are limited. The embryonic development of H. labeo was observed in the lower reaches of Fujiang River by He

[Fig. 1.] Map showing the sampling area in Goe-san Lake.

et al. (1999). Xu et al. (2007) studied the effects of food and temperature on the growth of H. labeo. Lv (2008) compared the morphological characters and their correlations in H. labeo that were 1-2 years old. Scale and growth characteristics were studied in H. labeo by Lv et al. (2008). In Korea, no study of the age and growth of this species has been previously conducted, although several studies have examined infection status (Kim et al., 2008) and cytogenetic characters for the species identification of Hemibarbus (Bang et al., 2008).

In this study, vertebrae were used to determine the age of H. labeo. The aim was to determine the age composition and pattern of the H. labeo population, and to estimate the growth based on age. Such measurements can provide essential data for the assessment of fish stocks (Hilborn and Walters, 1992), and would provide useful information and reference data for fish management and exploitation in Goe-san Lake. Such information will also be helpful for guiding the future culture of this species in Korea.

H. labeo were sampled from Goe-san Lake divided into upstream, midstream, and downstream areas (Fig. 1). Monthly samples from the lake were collected from March 2011 to February 2012, because the water in the lake was mostly frozen during January and February. In December and March, it was difficult to fish for barbel steel due to the low fish activities at lower temperatures. Fish were sampled by hook and line fishing, as well as from gill nets (length, 20 m; width, 1

[Fig. 2.] Hemibarbus labeo vertebrae showing measurements.

m; mesh size, 5.0 cm and 7.5 cm) in Goe-san Lake. In the field, the samples were kept in insulated cans with ice bags for transportation to the laboratory. In the laboratory, the specimens were measured (total length [TL]) and weighed (total weight [TW]). We macroscopically examined the gonads of both sexes of H. labeo from Goe-san Lake, based on color and size.

The relationship between TL and TW was determined by fitting the data to a potential relationship for males and females using the equation:

where TW is in grams, TL is in centimeters, and a and b are the parameters to be estimated.

The first to third vertebrae were removed and immersed in 8-10% potassium hydroxide for 24 h to remove the muscle and connective tissue. Then they were washed with running water, the remaining tissue was brushed out, and the vertebrae were fixed by immersion in 70% alcohol until further observation (Joung et al., 2005).

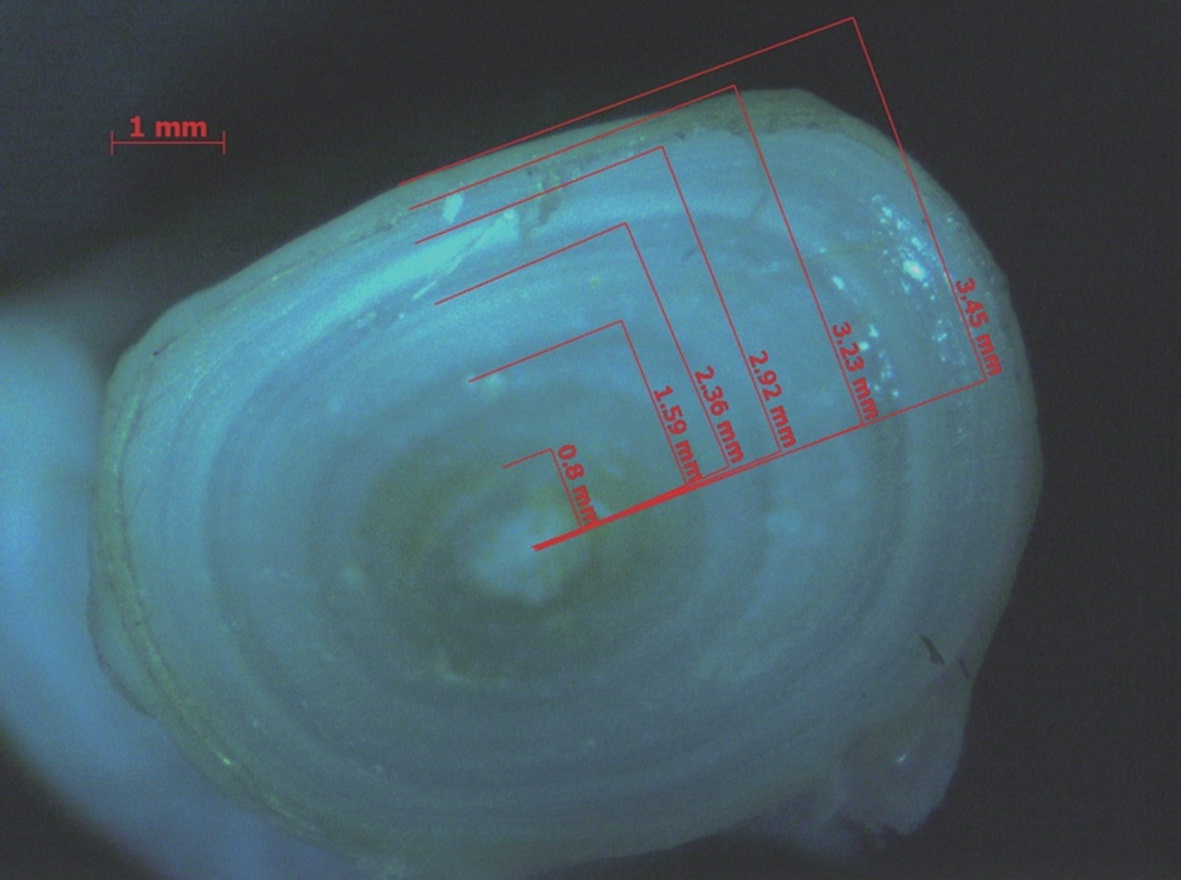
Ages were determined by counting the opaque zones in the vertebrae with an image processing system consisting of a computer, a video camera microscope (Zeiss DV8; Carl Zeiss, Jena, Germany) and the Optical Pattern Recognition System software package of Image-Pro Plus version 4.1 (Fig. 2).

Vertebrae were read twice at an interval of 20 days. They were read randomly to avoid bias in assigning ages. The average percentage error (APE) and coefficient of variation (CV) were used to compare age readings (Beamish and Fournier, 1981):

where R is the number of times each fish was aged, X_ij is the ith age determination of the jth fish, and X_i is the mean age calculated for the jth fish.

To validate the rings as indicators of fish age, rings were counted and radii were measured. The number of rings must show a directly proportional relation to vertebra size and fish length to be considered a growth indicator. This relationship was assessed by linear regression using the Fraser-Lee equation (Francis, 1990):

where R is the scale radius, and a and b are the parameters to be estimated.

Marginal increment (MI) analysis was used to validate the periodicity of growth (Lai et al., 1996):

where R represents the scale radius, and r_i and r_i-1 are the annular radii of the last and penultimate annuli, respectively. The period of annulus formation was considered that for which MI displayed its smallest value.

Measurements were used for back-calculating size from growth rings. Length at previous age was estimated based on the linear regression between TL and vertebral radius using the Fraser-Lee method (Natanson et al., 1995),

where [TL]_n is the back-calculated length at growth ring n, R_n is the vertebra radius at the time n, V_R is the vertebra radius at capture, TL is that at capture, and a is the intercept on the length axis of the linear relationship between TL and vertebra radius.

The growth curve was modeled using the von Bertalanffy growth equation:

where L_t is the TL at age t, L_∞ is asymptotic length, K is the coefficient of growth, and t₀ is the theoretical age when the predicted mean length is zero. The regression of fecundity on TL was analyzed. The ages at maturation by sex were calculated from this equation when the length at maturation was determined.

[Fig. 3.] Total length distribution in both sexes samples of Hemibarbus labeo.

Growth performance (φ') of a species can be captured by the growth index (Munro and Pauly, 1983). This value was used to compare the growth parameters obtained in the present work to those reported by others,

Length-weight relationships were tested for differences between sexes using analysis of covariance (ANCOVA). We used Kolmogorov-Smirnov tests (α = 0.05) to determine whether significant differences existed between males and females. Differences in MI during the months were evaluated by one-way analysis of variance (ANOVA). When the results of an ANOVA were significant, differences in the means of the variables were tested using the posterior Turkey-honestly significant difference method. A parametric paired t-test was used to compare assigned ages between two readings by one reader.

These statistical analyses were performed using the SPSS version 17.0 (SPSS Inc., Chicago, IL, USA). Significant differences were determined at the 0.05 probability level (P < 0.05) for all tests.

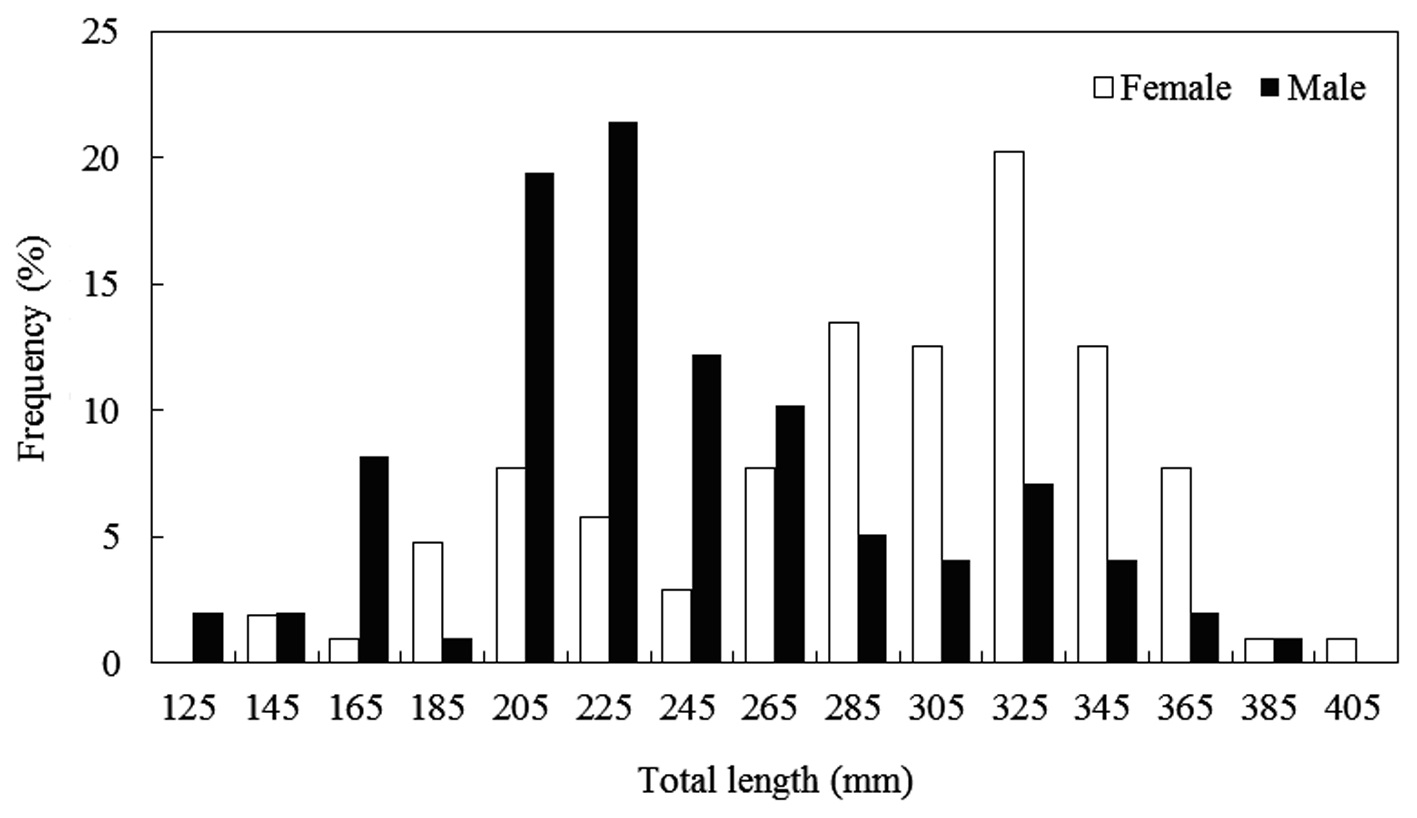
The caught H. labeo ranged in size from 125.0 mm to 421.0 mm in TL and from 11.65 g to 599.26 g in TW. Of the 201 specimens collected, 97 (48.26%) were identified as males, ranging from 125.0 to 387.3 mm and 14.65 g to 550.98 g, while the other 104 (51.74%) individuals were females from 150.0 mm to 421.0 mm and 28.72 g to 599.26 g (Table 1). TLs (± SD) of males and females were 250.7 (± 51.66) mm and 301.5 (± 46.92) mm, respectively. Females were larger than males. The length-frequency distribution is shown in Fig. 3. There was a significant difference in length-frequency distributions

[Table 1.] Composition of total length (mm) and total weight (g) of Hemibarbus labeo from April to November

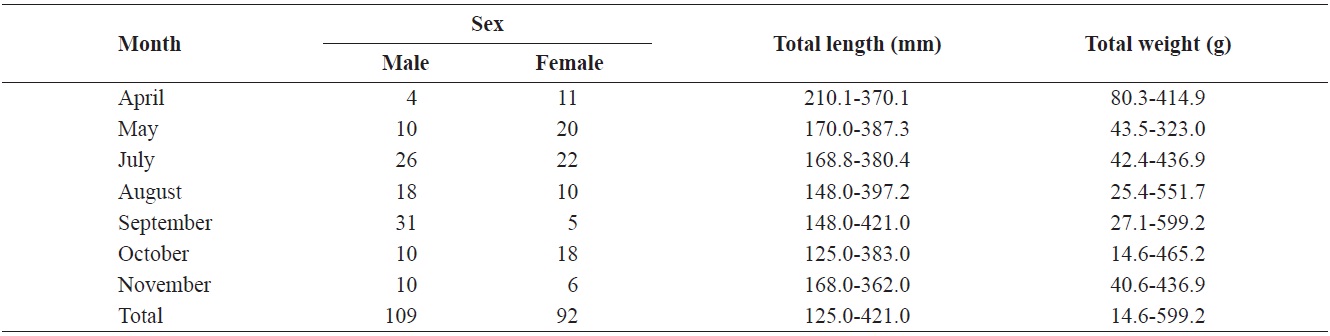
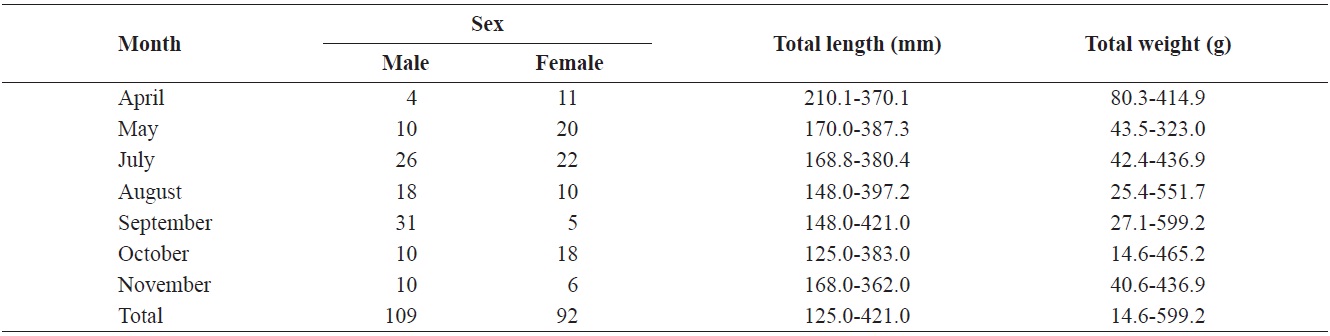
Composition of total length (mm) and total weight (g) of Hemibarbus labeo from April to November

between the two populations from upstream-midstream and downstream (d_max = 0.2035, P < 0.05).

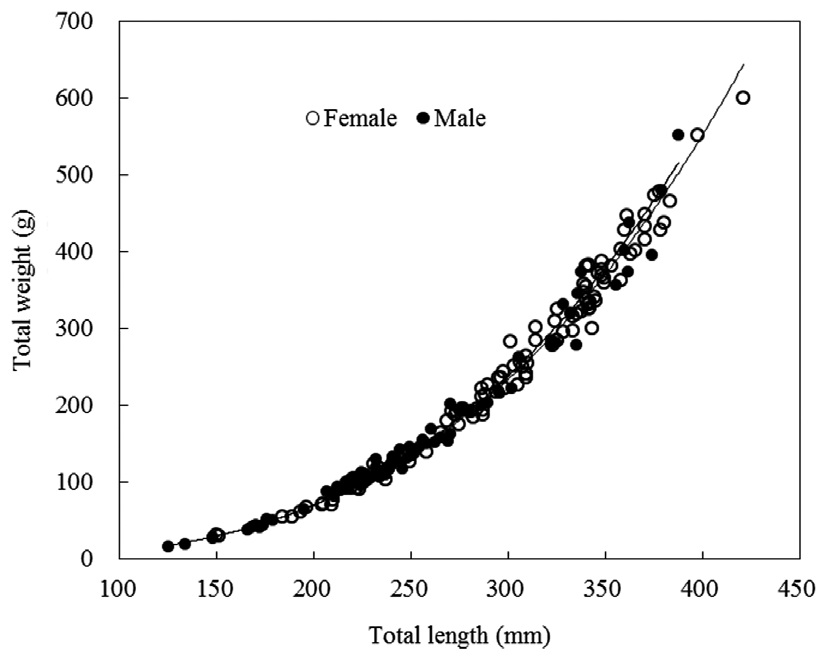
TW-TL relationships were separately evaluated for all individuals and grouped by sexes (females and males). The slopes

[Fig. 4.] Relationship of total length and total weight in the samples of Hemibarbus labeo.

[Fig. 5.] Monthly change of marginal growth index (MI) for male and female of Hemibarbus labeo.

of the TW-TL regressions did not differ significantly between sexes (ANCOVA test for equal slopes: F = 0.991, P > 0.05; ANCOVA test for intercepts: F = 15.68, P > 0.05). The regression equations were developed as TW = 9 × 10^-6 TL^2.987 (male) and TW = 8 × 10^-6 TL^3.014 (female) (Fig. 4). The equation for the combined sexes was TW = 9 × 10^-6 TL^2.988. The obtained values (b) were not significantly different from 3 (t-test, male: t = 1.35, P > 0.05; female: t = 2.01, P > 0.05), so the hypothesis of isometric growth was accepted for this species (Pauly, 1984).

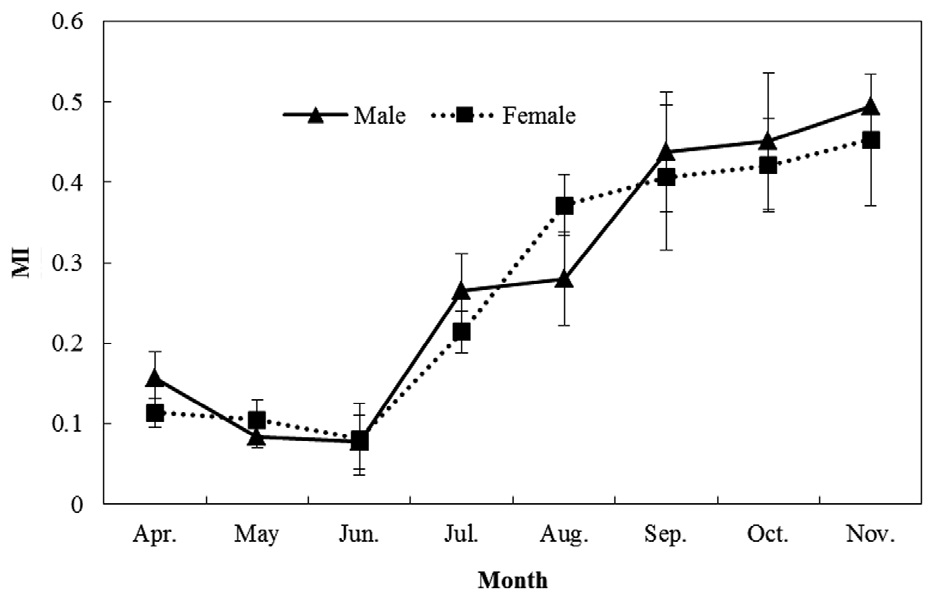
MI analysis (Fig. 5) showed a trend of increasing MI width from spring to autumn. Significant differences among months were found (one-way ANOVA for males: F = 14.44, P < 0.05; F = 21.78, P < 0.05). As shown in Fig. 4, the minimum increments in both sexes occurred in June, increased gradually from June to October, and attained the maximum value in October. Except for the MI value in winter, the rings formed annually, ad the same results have been found in some other freshwater fish including Ictalurus punctatus (Appelget and Smith, 1951), Glyptosternon maculatum (Ding et al., 2011), Silurus glanis (Alp et al., 2011), and Schizothorax o’connori (Ma et al., 2011). Therefore, annual ring formation appears to be completed during June to July in Goe-san Lake.

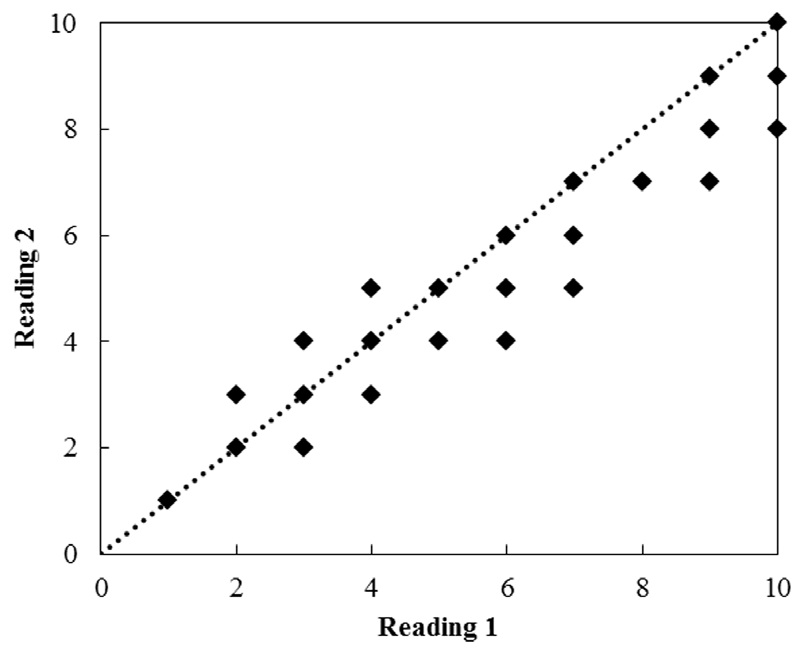
Age estimates ranged from 2 to 10 years for females and from 1 to 8 years for males, based on the examination of 201 vertebral centers. Each vertebra was read twice (Fig. 6). The APE estimated from the two readings ranged from 0.02 to 0.39 (mean, 0.18), and the CV ranged from 0.04 to 0.78 (mean, 0.23). The paired t-test applied to compare the age assigned by the two readings revealed significant differences (P < 0.05) (Fig. 6). The older the fish, the greater the bias.

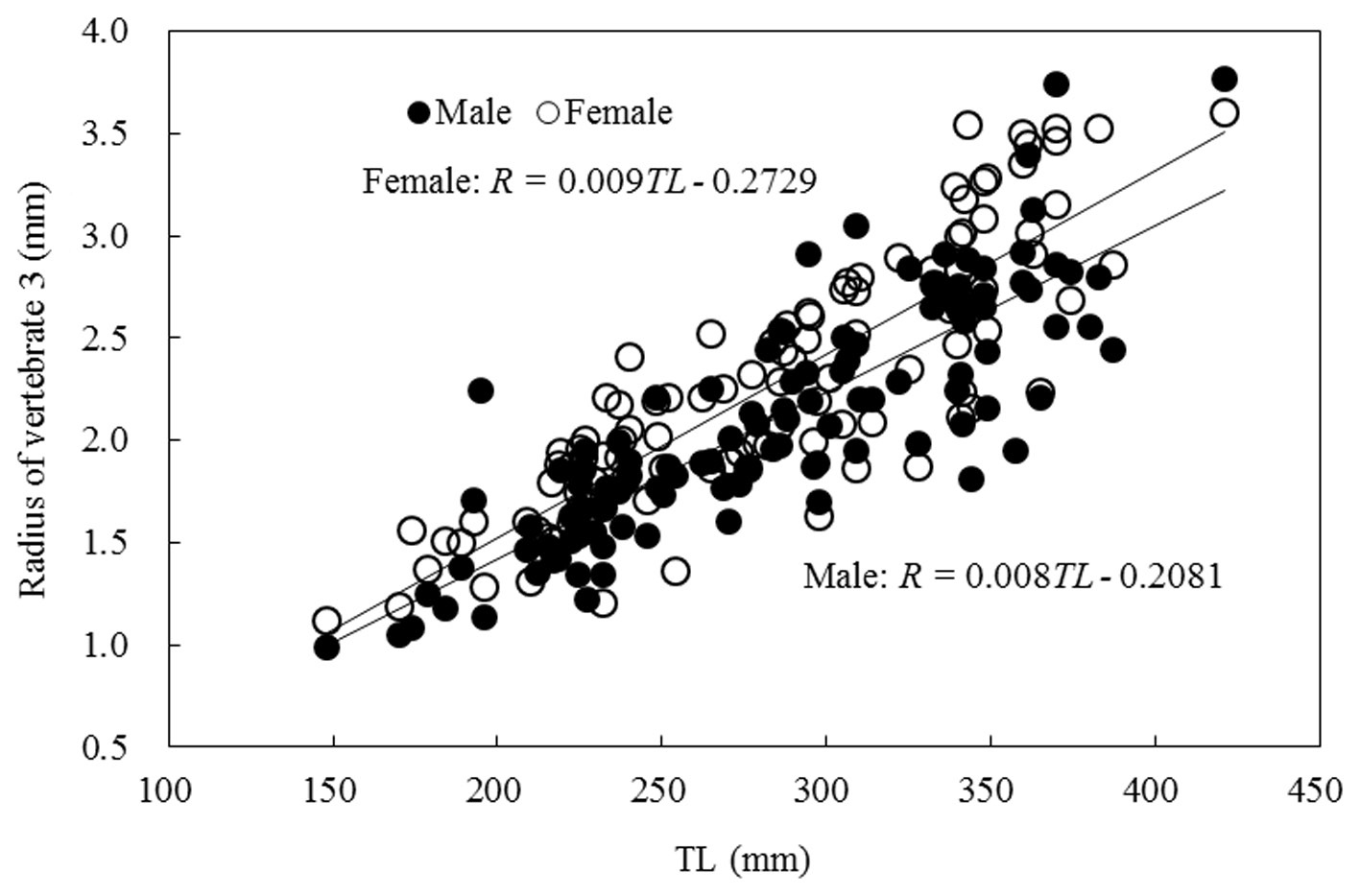
The relationship between the TL and vertebral radius was

[Fig. 6.] Age bias plot for reconciled age estimates from the reading 1 and reading 2 of vertebrae of Hemibarbus labeo (combined sexes, n = 147) in the Goe-san Lake. The dash line is the 45° equivalence slope.

[Fig. 7.] Regression linear of total length (TL) and vertebrae radius of Hemibarbus labeo for male and female.

[Fig. 8.] The von Bertalanffy growth curves estimated by vertebra reading of Hemibabus labeo for male, female and combined sex. TL, total length.

linear, as shown in Fig. 7, and was expressed as follows:

Male: R = 0.008TL - 0.208 (r2 = 0.823)

Female: R = 0.009TL - 0.272 (r2 = 0.835).

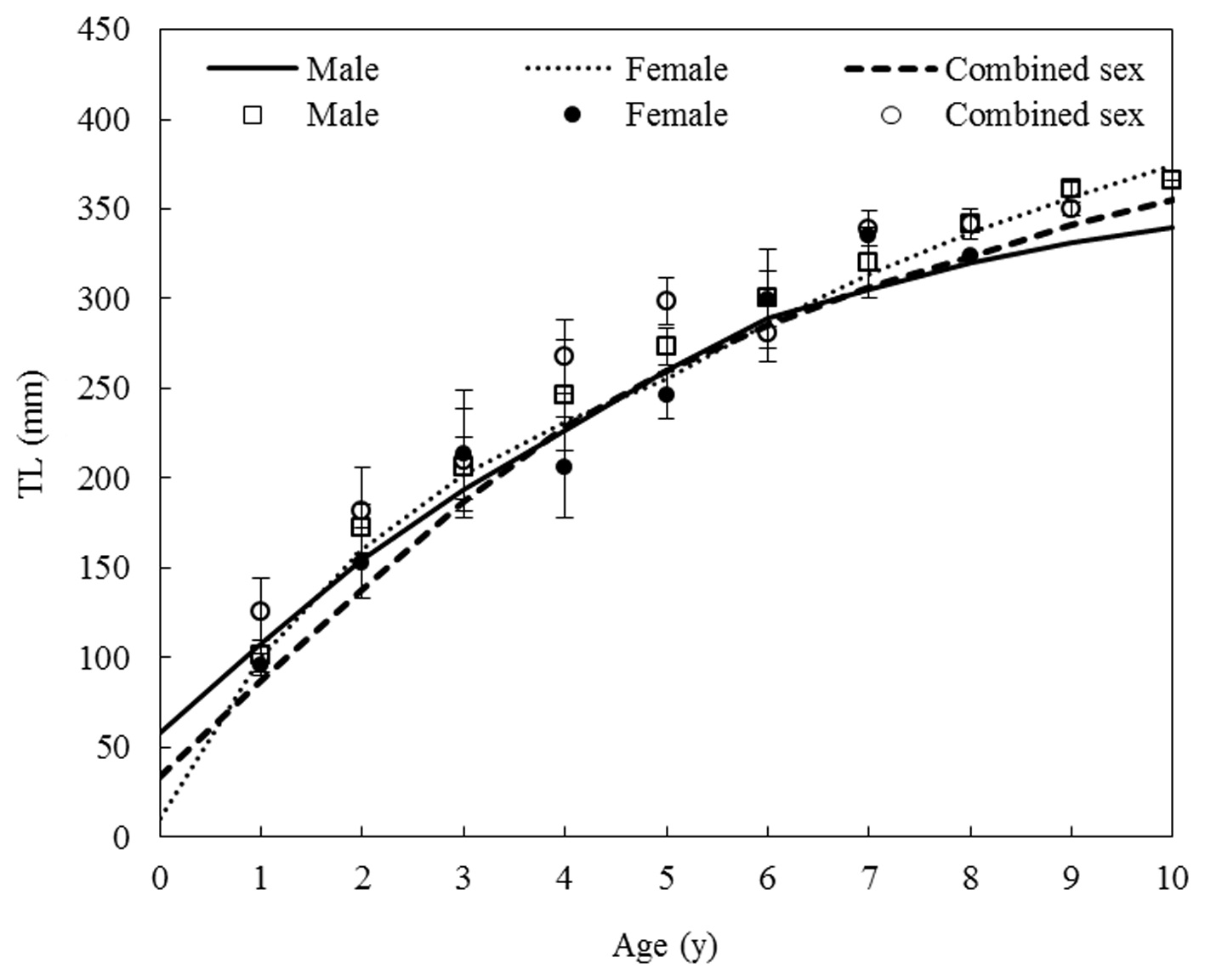
Back-calculated TLs were obtained from the corrected ring radius using the Fraser-Lee method, which gives the TL at the time of ring formation for males, females, and combined sexes. There was no indication of Rose Lee’s phenomenon, in which computed sizes at a given age tend to be smaller when derived from measurements of older fish (Francis, 1990).

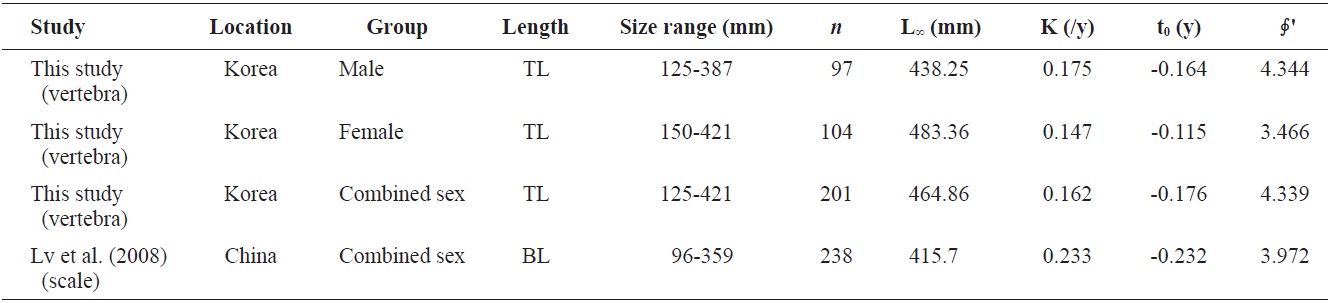
Von Bertalanffy growth equations were determined from the back-calculated TLs of males, females, and combined sexes. The equations were L_t = 438.25(1 - e^{-0.175(t+0.164)}), for males, L_t = 483.36(1 - e^{-0.147(t+0.115)}) for females, and L_t = 464.86(1 - e^{-0.162(t+0.176)}) for the sexes combined (Fig. 8). The growth performances were 4.526, 4.536, and 4.544, respectively. There was no significant difference in the von Bertalanffy growth curves (F-test, P > 0.05) between sexes.

This study reports on the age and growth of H. labeo in Goe-san Lake, using vertebrae as an age determinant marker. Katsanevakis and Maravelias (2008) stated that the choice of the best growth model is subjective and should be in some cases based on the decision of the researcher, founded on experience with the species and previous studies to interpret the viability of estimated parameters and goodness-of-fit. Cailliet and Goldman (2004) mentioned that there has been an increase in the use of both verification and validation methodologies in fish growth. Using a combination of verification and validation approaches is most likely to produce convincing results. In this study, scales and vertebrae could have been used to determine fish age by comparison with other calcified structures in the authors experience, but Lv et al. (2008) studied the growth of H. labeo from scales. Therefore, we chose to use vertebrae as a comparison.

Vertebral rings occur systematically as length increases. Their formation is probably more directly related to factors other than size (Pratt and Casey, 1983). All of the factors influencing the formation of the opaque zone are not clear, but several hypotheses explaining its deposition have been reported. Food shortages and food deprivation caused by migration and spawning (Yosef and Casselman, 1995) may affect zone formation. In this study, the water flow rate of a dam directly affected an increase in food availability in March and April,

[Table 2.] Growth parameters of Hemibarbus labeo (male, female, and combined sexes) from this study and other studies

Growth parameters of Hemibarbus labeo (male, female, and combined sexes) from this study and other studies

corresponding to the time of opaque band formation, suggesting that the fast growth of H. labeo might be correlated to its feeding behavior. However, the underlying mechanisms governing band deposition still need to be determined.

In this study, H. labeo grew relatively fast during the first 3 years of life, and attained about 40% of maximum length during the third year. The highest growth rate occurred in the first year (Fig. 8). After the second year, the annual growth rate dropped rapidly. This is consistent with the results of Lv et al. (2008), and may be related to physiological changes caused by factors such as food availability, temperature, and sexual maturity.

The growth performance of fish reflects prevailing abiotic conditions such as temperature regime, as well as the ability of a species to meet its nutritional requirements (Beamesderfer and North, 1995). This value was also used to compare the growth parameters obtained in the present work to those reported by others. A comparison of the parameters of von Bertalanffy growth equations from the literature (Table 2) showed that male H. labeo have higher growth potential than females in Goe-san Lake, and that the growth potential of H. labeo collected from the Wusulijiang River in China us lower than that of H. labeo collected from Goe-san Lake. This may be related to the good conditions in this lake, which is not overexploited.